The Formation of Complex Ions

17.3 The Formation of Complex Ions

Learning Objective

To describe the formation of complex ions quantitatively.

In Chapter 4 "Reactions in Aqueous Solution", you learned that metal ions in aqueous solution are hydrated—that is, surrounded by a shell of usually four or six water molecules. A hydrated ion is one kind of a complex ionAn ionic species that forms between a central metal ion and one or more surrounding ligands because of a Lewis acid–base interaction. The positively charged metal ion acts as the Lewis acid, and the ligand acts as the Lewis base. (or, simply, complex), a species formed between a central metal ion and one or more surrounding ligandsAn ion or a molecule that contains one or more pairs of electrons that can be shared with the central metal in a metal complex., molecules or ions that contain at least one lone pair of electrons, such as the [Al(H₂O)₆]³⁺ ion in Figure 16.12 "Effect of a Metal Ion on the Acidity of Water".

A complex ion forms from a metal ion and a ligand because of a Lewis acid–base interaction. The positively charged metal ion acts as a Lewis acid, and the ligand, with one or more lone pairs of electrons, acts as a Lewis base. Small, highly charged metal ions, such as Cu²⁺ or Ru³⁺, have the greatest tendency to act as Lewis acids, and consequently, they have the greatest tendency to form complex ions.

As an example of the formation of complex ions, consider the addition of ammonia to an aqueous solution of the hydrated Cu²⁺ ion {[Cu(H₂O)₆]²⁺}. Because it is a stronger base than H₂O, ammonia replaces the water molecules in the hydrated ion to form the [Cu(NH₃)₄(H₂O)₂]²⁺ ion. Formation of the [Cu(NH₃)₄(H₂O)₂]²⁺ complex is accompanied by a dramatic color change, as shown in Figure 17.4 "The Formation of Complex Ions". The solution changes from the light blue of [Cu(H₂O)₆]²⁺ to the blue-violet characteristic of the [Cu(NH₃)₄(H₂O)₂]²⁺ ion.

Figure 17.4 The Formation of Complex Ions

An aqueous solution of CuSO₄ consists of hydrated Cu²⁺ ions in the form of pale blue [Cu(H₂O)₆]²⁺ (left). The addition of aqueous ammonia to the solution results in the formation of the intensely blue-violet [Cu(NH₃)₄(H₂O)₂]²⁺ ions, usually written as [Cu(NH₃)₄]²⁺ ion (right) because ammonia, a stronger base than H₂O, replaces water molecules from the hydrated Cu²⁺ ion.

The Formation Constant

The replacement of water molecules from [Cu(H₂O)₆]²⁺ by ammonia occurs in sequential steps. Omitting the water molecules bound to Cu²⁺ for simplicity, we can write the equilibrium reactions as follows:

Equation 17.6

\begin{matrix} {Cu}^{2 +} (aq) + {NH}_{3} (aq) & ⇌ {[Cu ({NH}_{3})]}^{2 +} (aq) & K_{1} \\ {[Cu ({NH}_{3})]}^{2 +} (aq) + {NH}_{3} (aq) & ⇌ {[Cu {({NH}_{3})}_{2}]}^{2 +} (aq) & K_{2} \\ {[Cu {({NH}_{3})}_{2}]}^{2 +} (aq) + {NH}_{3} (aq) & ⇌ {[Cu {({NH}_{3})}_{3}]}^{2 +} (aq) & K_{3} \\ {[Cu {({NH}_{3})}_{3}]}^{2 +} (aq) + {NH}_{3} (aq) & ⇌ {[Cu {({NH}_{3})}_{4}]}^{2 +} (aq) & K_{4} \end{matrix}

The sum of the stepwise reactions is the overall equation for the formation of the complex ion:The hydrated Cu²⁺ ion contains six H₂O ligands, but the complex ion that is produced contains only four NH₃ ligands, not six. The reasons for this apparently unusual behavior will be discussed in Chapter 23 "The ".

Equation 17.7

{Cu}^{2 +} (aq) + {4NH}_{3} (aq) ⇌ {[Cu {({NH}_{3})}_{4}]}^{2 +} (aq)

The equilibrium constant for the formation of the complex ion from the hydrated ion is called the formation constant (K_f)The equilibrium constant for the formation of a complex ion from a hydrated metal ion; that is, for the reaction $a A + b B ⇌ c C + d D,$ $K_{f} = {[C]}^{c} {[D]}^{d} / {[A]}^{a} {[B]}^{b} .$ . The equilibrium constant expression for K_f has the same general form as any other equilibrium constant expression. In this case, the expression is as follows:

Equation 17.8

K_{f} = \frac{[{[Cu {({NH}_{3})}_{4}]}^{2 +}]}{[{Cu}^{2 +}] {[{NH}_{3}]}^{4}} = 2.1 \times 10^{13} = K_{1} K_{2} K_{3} K_{4}

Note the Pattern

The formation constant (K_f) has the same general form as any other equilibrium constant expression.

Water, a pure liquid, does not appear explicitly in the equilibrium constant expression, and the hydrated Cu²⁺(aq) ion is represented as Cu²⁺ for simplicity. As for any equilibrium, the larger the value of the equilibrium constant (in this case, K_f), the more stable the product. With K_f = 2.1 × 10¹³, the [Cu(NH₃)₄(H₂O)₂]²⁺ complex ion is very stable. The formation constants for some common complex ions are listed in Table 17.2 "Formation Constants for Selected Complex Ions in Aqueous Solution*".

Table 17.2 Formation Constants for Selected Complex Ions in Aqueous Solution*

	Complex Ion	Equilibrium Equation	K _f
Ammonia Complexes	[Ag(NH₃)₂]⁺	${Ag}^{+} + 2 {NH}_{3} ⇌ {[Ag {({NH}_{3})}_{2}]}^{+}$	1.1 × 10⁷
	[Cu(NH₃)₄]²⁺	${Cu}^{2 +} + {4NH}_{3} ⇌ {[Cu {({NH}_{3})}_{4}]}^{2 +}$	2.1 × 10¹³
	[Ni(NH₃)₆]²⁺	${Ni}^{2 +} + 6 {NH}_{3} ⇌ {[Ni {({NH}_{3})}_{6}]}^{2 +}$	5.5 × 10⁸
Cyanide Complexes	[Ag(CN)₂]⁻	${Ag}^{+} + 2 {CN}^{-} ⇌ {[{Ag(CN)}_{2}]}^{-}$	1.1 × 10¹⁸
	[Ni(CN)₄]²⁻	${Ni}^{2 +} + 4 {CN}^{-} ⇌ {[{Ni(CN)}_{4}]}^{2 -}$	2.2 × 10³¹
	[Fe(CN)₆]³⁻	${Fe}^{3 +} + 6 {CN}^{-} ⇌ {[{Fe(CN)}_{6}]}^{3 -}$	1 × 10⁴²
Hydroxide Complexes	[Zn(OH)₄]²⁻	${Zn}^{2 +} + 4 {OH}^{-} ⇌ {[{Zn(OH)}_{4}]}^{2 -}$	4.6 × 10¹⁷
Hydroxide Complexes	[Cr(OH)₄]⁻	${Cr}^{3 +} + 4 {OH}^{-} ⇌ {[{Cr(OH)}_{4}]}^{-}$	8.0 × 10²⁹
Halide Complexes	[HgCl₄]²⁻	${Hg}^{2 +} + 4 {Cl}^{-} ⇌ {[{HgCl}_{4}]}^{2 -}$	1.2 × 10¹⁵
	[CdI₄]²⁻	${Cd}^{2 +} + 4 I ⇌ {[{CdI}_{4}]}^{2 -}$	2.6 × 10⁵
	[AlF₆]³⁻	${Al}^{3 +} + 6 F^{-} ⇌ {[{AlF}_{6}]}^{3 -}$	6.9 × 10¹⁹
Other Complexes	[Ag(S₂O₃)₂]³⁻	${Ag}^{+} + 2 S_{2} O_{3}^{2 -} ⇌ {[{Ag(S}_{2} O_{3})_{2}]}^{3 -}$	2.9 × 10¹³
Other Complexes	[Fe(C₂O₄)₃]³⁻	${Fe}^{3 +} + 3 C_{2} O_{4}^{2 -} ⇌ {[Fe {(C_{2} O_{4})}_{3}]}^{3 -}$	2.0 × 10²⁰
*Reported values are overall formation constants.

Source: Data from Lange’s Handbook of Chemistry, 15th ed. (1999).

Example 5

If 12.5 g of Cu(NO₃)₂·6H₂O is added to 500 mL of 1.00 M aqueous ammonia, what is the equilibrium concentration of Cu²⁺(aq)?

Given: mass of Cu²⁺ salt and volume and concentration of ammonia solution

Asked for: equilibrium concentration of Cu²⁺(aq)

Strategy:

A Calculate the initial concentration of Cu²⁺ due to the addition of copper(II) nitrate hexahydrate. Use the stoichiometry of the reaction shown in Equation 17.7 to construct a table showing the initial concentrations, the changes in concentrations, and the final concentrations of all species in solution.

B Substitute the final concentrations into the expression for the formation constant (Equation 17.8) to calculate the equilibrium concentration of Cu²⁺(aq).

Solution:

Adding an ionic compound that contains Cu²⁺ to an aqueous ammonia solution will result in the formation of [Cu(NH₃)₄]²⁺(aq), as shown in Equation 17.7. We assume that the volume change caused by adding solid copper(II) nitrate to aqueous ammonia is negligible.

A The initial concentration of Cu²⁺ from the amount of added copper nitrate prior to any reaction is as follows:

12.5 g Cu {({NO}_{3})}_{2} • 6 H_{2} O (\frac{1 mol}{295.65 g}) (\frac{1}{500 mL}) (\frac{1000 mL}{1 L}) = 0.0846 M

Because the stoichiometry of the reaction is four NH₃ to one Cu²⁺, the amount of NH₃ required to react completely with the Cu²⁺ is 4(0.0846) = 0.338 M. The concentration of ammonia after complete reaction is 1.00 M − 0.338 M = 0.66 M. These results are summarized in the first two lines of the following table. Because the equilibrium constant for the reaction is large (2.1 × 10¹³), the equilibrium will lie far to the right. Thus we will assume that the formation of [Cu(NH₃)₄]²⁺ in the first step is complete and allow some of it to dissociate into Cu²⁺ and NH₃ until equilibrium has been reached. If we define x as the amount of Cu²⁺ produced by the dissociation reaction, then the stoichiometry of the reaction tells us that the change in the concentration of [Cu(NH₃)₄]²⁺ is −x, and the change in the concentration of ammonia is +4x, as indicated in the table. The final concentrations of all species (in the bottom row of the table) are the sums of the concentrations after complete reaction and the changes in concentrations.

${Cu}^{2 +} + 4 {NH}_{3} ⇌ {[Cu {({NH}_{3})}_{4}]}^{2 +}$
	[Cu²⁺]	[NH₃]	[[Cu(NH₃)₄]²⁺]
initial	0.0846	1.00	0
after complete reaction	0	0.66	0.0846
change	+x	+4x	−x
final	x	0.66 + 4x	0.0846 − x

B Substituting the final concentrations into the expression for the formation constant (Equation 17.8) and assuming that x << 0.0846, which allows us to remove x from the sum and difference,

\begin{matrix} K_{f} & = \frac{[{[Cu {({NH}_{3})}_{4}]}^{2 +}]}{[{Cu}^{2 +}] {[{NH}_{3}]}^{4}} = \frac{0.0846 - x}{x {(0.66 + 4 x)}^{4}} \approx \frac{0.0846}{x {(0.66)}^{4}} = 2.1 \times 10^{13} \\ x & = 2.1 \times 10^{- 14} \end{matrix}

The value of x indicates that our assumption was justified. The equilibrium concentration of Cu²⁺(aq) in a 1.00 M ammonia solution is therefore 2.1 × 10⁻¹⁴ M.

Exercise

The ferrocyanide ion {[Fe(CN)₆]⁴⁻} is very stable, with a K_f of 1 × 10³⁵. Calculate the concentration of cyanide ion in equilibrium with a 0.65 M solution of K₄[Fe(CN)₆].

Answer: 2 × 10⁻⁶ M

The Effect of the Formation of Complex Ions on Solubility

What happens to the solubility of a sparingly soluble salt if a ligand that forms a stable complex ion is added to the solution? One such example occurs in conventional black-and-white photography, which was discussed briefly in Chapter 4 "Reactions in Aqueous Solution".

Recall that black-and-white photographic film contains light-sensitive microcrystals of AgBr, or mixtures of AgBr and other silver halides. AgBr is a sparingly soluble salt, with a K_sp of 5.35 × 10⁻¹³ at 25°C. When the shutter of the camera opens, the light from the object being photographed strikes some of the crystals on the film and initiates a photochemical reaction that converts AgBr to black Ag metal. Well-formed, stable negative images appear in tones of gray, corresponding to the number of grains of AgBr converted, with the areas exposed to the most light being darkest. To fix the image and prevent more AgBr crystals from being converted to Ag metal during processing of the film, the unreacted AgBr on the film is removed using a complexation reaction to dissolve the sparingly soluble salt.

The reaction for the dissolution of silver bromide is as follows:

Equation 17.9

\begin{matrix} AgBr(s) ⇌ {Ag}^{+} (aq) + {Br}^{-} (aq) & K_{sp} = 5.35 \times 10^{- 13} at 25°C \end{matrix}

The equilibrium lies far to the left, and the equilibrium concentrations of Ag⁺ and Br⁻ ions are very low (7.31 × 10⁻⁷ M). As a result, removing unreacted AgBr from even a single roll of film using pure water would require tens of thousands of liters of water and a great deal of time. Le Châtelier’s principle tells us, however, that we can drive the reaction to the right by removing one of the products, which will cause more AgBr to dissolve. Bromide ion is difficult to remove chemically, but silver ion forms a variety of stable two-coordinate complexes with neutral ligands, such as ammonia, or with anionic ligands, such as cyanide or thiosulfate (S₂O₃²⁻). In photographic processing, excess AgBr is dissolved using a concentrated solution of sodium thiosulfate.

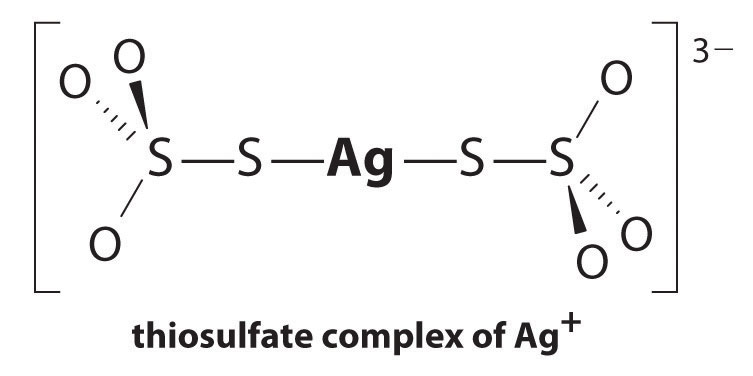
The reaction of Ag⁺ with thiosulfate is as follows:

Equation 17.10

\begin{matrix} {Ag}^{+} (aq) + 2 S_{2} O_{3}^{2 -} (aq) ⇌ {[Ag {(S_{2} O_{3})}_{2}]}^{3 -} (aq) & K_{f} = 2.9 \times 10^{13} \end{matrix}

The magnitude of the equilibrium constant indicates that almost all Ag⁺ ions in solution will be immediately complexed by thiosulfate to form [Ag(S₂O₃)₂]³⁻. We can see the effect of thiosulfate on the solubility of AgBr by writing the appropriate reactions and adding them together:

Equation 17.11

\begin{matrix} AgBr(s) ⇌ {Ag}^{+} (aq) + {Br}^{-} (aq) & K_{sp} & = 5.35 \times 10^{- 13} \\ {Ag}^{+} (aq) + 2 S_{2} O_{3}^{2 -} (aq) ⇌ {[Ag {(S_{2} O_{3})}_{2}]}^{3 -} (aq) & K_{f} & = 2.9 \times 10^{13} \\ AgBr(s) + 2 S_{2} O_{3}^{2 -} (aq) ⇌ {[Ag {(S_{2} O_{3})}_{2}]}^{3 -} (aq) + {Br}^{-} (aq) & K & = K_{sp} K_{f} = 15 \end{matrix}

Comparing K with K_sp shows that the formation of the complex ion increases the solubility of AgBr by approximately 3 × 10¹³. The dramatic increase in solubility combined with the low cost and the low toxicity explains why sodium thiosulfate is almost universally used for developing black-and-white film. If desired, the silver can be recovered from the thiosulfate solution using any of several methods and recycled.

Note the Pattern

If a complex ion has a large K_f, the formation of a complex ion can dramatically increase the solubility of sparingly soluble salts.

Example 6

Due to the common ion effect, we might expect a salt such as AgCl to be much less soluble in a concentrated solution of KCl than in water. Such an assumption would be incorrect, however, because it ignores the fact that silver ion tends to form a two-coordinate complex with chloride ions (AgCl₂⁻). Calculate the solubility of AgCl in each situation:

in pure water
in 1.0 M KCl solution, ignoring the formation of any complex ions
the same solution as in part (b) except taking the formation of complex ions into account, assuming that AgCl₂⁻ is the only Ag⁺ complex that forms in significant concentrations

At 25°C, K_sp = 1.77 × 10⁻¹⁰ for AgCl and K_f = 1.1 × 10⁵ for AgCl₂⁻.

Given: K_sp of AgCl, K_f of AgCl₂⁻, and KCl concentration

Asked for: solubility of AgCl in water and in KCl solution with and without the formation of complex ions

Strategy:

A Write the solubility product expression for AgCl and calculate the concentration of Ag⁺ and Cl⁻ in water.

B Calculate the concentration of Ag⁺ in the KCl solution.

C Write balanced chemical equations for the dissolution of AgCl and for the formation of the AgCl₂⁻ complex. Add the two equations and calculate the equilibrium constant for the overall equilibrium.

D Write the equilibrium constant expression for the overall reaction. Solve for the concentration of the complex ion.

Solution:

A If we let x equal the solubility of AgCl, then at equilibrium [Ag⁺] = [Cl⁻] = x M. Substituting this value into the solubility product expression,
$\begin{matrix} K_{sp} = & [{Ag}^{+}] [{Cl}^{-}] & = (x) (x) = x^{2} = 1.77 \times 10^{- 10} \\ x & = 1.33 \times 10^{- 5} \end{matrix}$
Thus the solubility of AgCl in pure water at 25°C is 1.33 × 10⁻⁵ M.
B If x equals the solubility of AgCl in the KCl solution, then at equilibrium [Ag⁺] = x M and [Cl⁻] = (1.0 + x) M. Substituting these values into the solubility product expression and assuming that x << 1.0,
$K_{sp} = [{Ag}^{+}] [{Cl}^{-}] = (x) (1.0 + x) \approx x (1.0) = 1.77 \times 10^{- 10} = x$
If the common ion effect were the only important factor, we would predict that AgCl is approximately five orders of magnitude less soluble in a 1.0 M KCl solution than in water.
C To account for the effects of the formation of complex ions, we must first write the equilibrium equations for both the dissolution and the formation of complex ions. Adding the equations corresponding to K_sp and K_f gives us an equation that describes the dissolution of AgCl in a KCl solution. The equilibrium constant for the reaction is therefore the product of K_sp and K_f:
$\begin{matrix} AgCl(s) ⇌ {Ag}^{+} (aq) + {Cl}^{-} (aq) & K_{sp} & = 1.77 \times 10^{- 10} \\ {Ag}^{+} (aq) + 2 {Cl}^{-} ⇌ {[{AgCl}_{2}]}^{-} & K_{f} & = 1.1 \times 10^{5} \\ AgCl(s) + {Cl}^{-} ⇌ {[{AgCl}_{^{2}}]}^{-} & K & = K_{sp} K_{f} = 1.9 \times 10^{- 5} \end{matrix}$
D If we let x equal the solubility of AgCl in the KCl solution, then at equilibrium [AgCl₂⁻] = x and [Cl⁻] = 1.0 − x. Substituting these quantities into the equilibrium constant expression for the net reaction and assuming that x << 1.0,
$K = \frac{[{AgCl}_{2}^{-}]}{[{Cl}^{-}]} = \frac{x}{1.0 - x} \approx 1.9 \times 10^{- 5} = x$
That is, AgCl dissolves in 1.0 M KCl to produce a 1.9 × 10⁻⁵ M solution of the AgCl₂⁻ complex ion. Thus we predict that AgCl has approximately the same solubility in a 1.0 M KCl solution as it does in pure water, which is 10⁵ times greater than that predicted based on the common ion effect. (In fact, the measured solubility of AgCl in 1.0 M KCl is almost a factor of 10 greater than that in pure water, largely due to the formation of other chloride-containing complexes.)

Exercise

Calculate the solubility of mercury(II) iodide (HgI₂) in each situation:

pure water
a 3.0 M solution of NaI, assuming [HgI₄]²⁻ is the only Hg-containing species present in significant amounts

K_sp = 2.9 × 10⁻²⁹ for HgI₂ and K_f = 6.8 × 10²⁹ for [HgI₄]²⁻.

Answer:

1.9 × 10⁻¹⁰ M
1.4 M

Complexing agents, molecules or ions that increase the solubility of metal salts by forming soluble metal complexes, are common components of laundry detergents. Long-chain carboxylic acids, the major components of soaps, form insoluble salts with Ca²⁺ and Mg²⁺, which are present in high concentrations in “hard” water. The precipitation of these salts produces a bathtub ring and gives a gray tinge to clothing. Adding a complexing agent such as pyrophosphate (O₃POPO₃⁴⁻, or P₂O₇⁴⁻) or triphosphate (P₃O₁₀⁵⁻) to detergents prevents the magnesium and calcium salts from precipitating because the equilibrium constant for complex-ion formation is large:

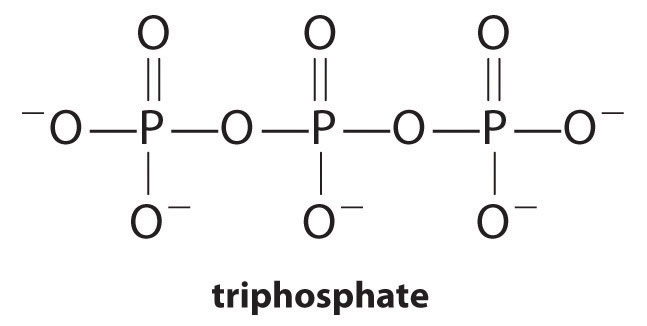
Equation 17.12

\begin{matrix} {Ca}^{2 +} (aq) + O_{3} {POPO}_{3}^{4 -} (aq) ⇌ {[Ca (O_{3} {POPO}_{3})]}^{2 -} (aq) & K_{f} = 4 \times 10^{4} \end{matrix}

However, phosphates can cause environmental damage by promoting eutrophication, the growth of excessive amounts of algae in a body of water, which can eventually lead to large decreases in levels of dissolved oxygen that kill fish and other aquatic organisms. Consequently, many states in the United States have banned the use of phosphate-containing detergents, and France has banned their use beginning in 2007. “Phosphate-free” detergents contain different kinds of complexing agents, such as derivatives of acetic acid or other carboxylic acids. The development of phosphate substitutes is an area of intense research.

Commercial water softeners also use a complexing agent to treat hard water by passing the water over ion-exchange resins, which are complex sodium salts. When water flows over the resin, sodium ion is dissolved, and insoluble salts precipitate onto the resin surface. Water treated in this way has a saltier taste due to the presence of Na⁺, but it contains fewer dissolved minerals.

Another application of complexing agents is found in medicine. Unlike x-rays, magnetic resonance imaging (MRI) can give relatively good images of soft tissues such as internal organs. MRI is based on the magnetic properties of the ¹H nucleus of hydrogen atoms in water, which is a major component of soft tissues. Because the properties of water do not depend very much on whether it is inside a cell or in the blood, it is hard to get detailed images of these tissues that have good contrast. To solve this problem, scientists have developed a class of metal complexes known as “MRI contrast agents.” Injecting an MRI contrast agent into a patient selectively affects the magnetic properties of water in cells of normal tissues, in tumors, or in blood vessels and allows doctors to “see” each of these separately (Figure 17.5 "An MRI Image of the Heart, Arteries, and Veins"). One of the most important metal ions for this application is Gd³⁺, which with seven unpaired electrons is highly paramagnetic. Because Gd³⁺(aq) is quite toxic, it must be administered as a very stable complex that does not dissociate in the body and can be excreted intact by the kidneys. The complexing agents used for gadolinium are ligands such as DTPA⁵⁻ (diethylene triamine pentaacetic acid), whose fully protonated form is shown here.

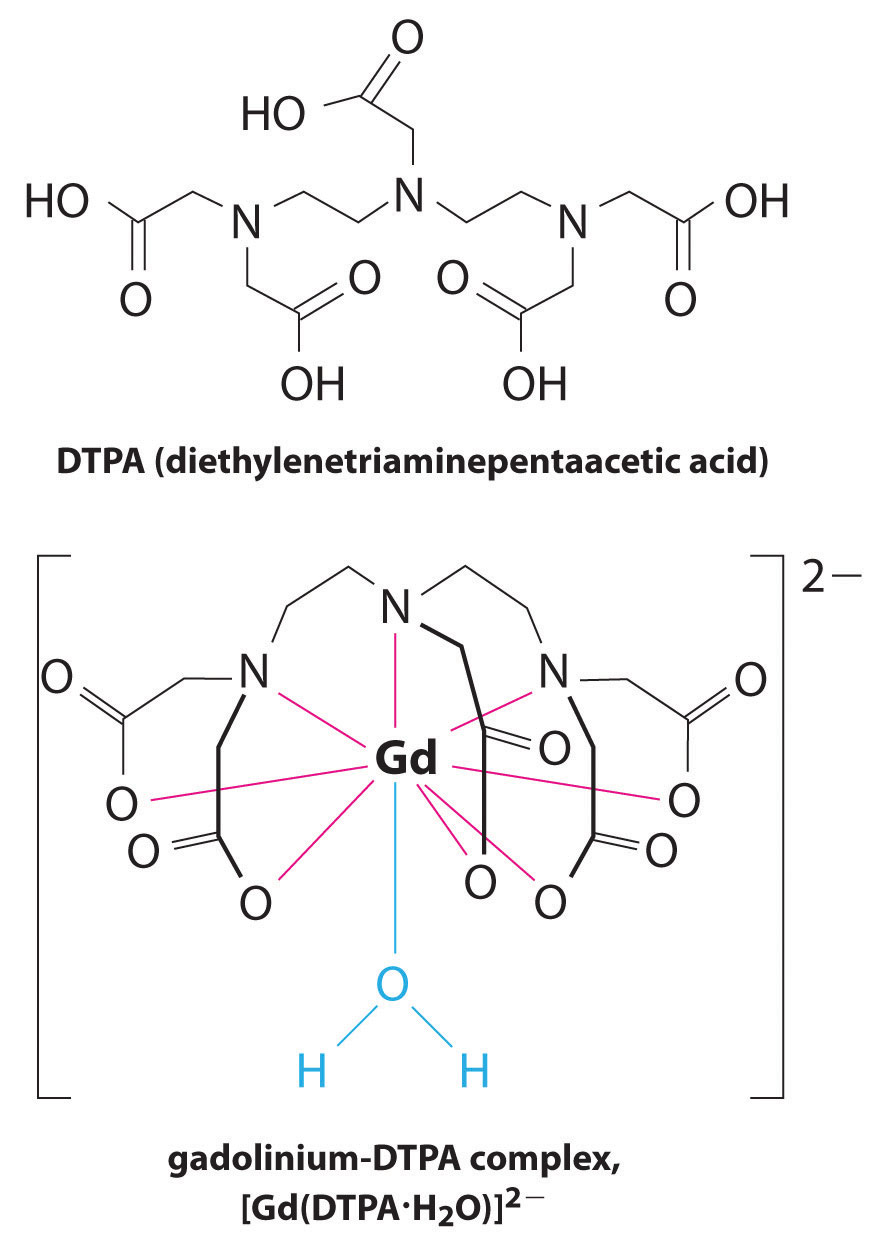
Figure 17.5 An MRI Image of the Heart, Arteries, and Veins

When a patient is injected with a paramagnetic metal cation in the form of a stable complex known as an MRI contrast agent, the magnetic properties of water in cells are altered. Because the different environments in different types of cells respond differently, a physician can obtain detailed images of soft tissues.

Summary

A complex ion is a species formed between a central metal ion and one or more surrounding ligands, molecules or ions that contain at least one lone pair of electrons. Small, highly charged metal ions have the greatest tendency to act as Lewis acids and form complex ions. The equilibrium constant for the formation of the complex ion is the formation constant (K_f). The formation of a complex ion by adding a complexing agent increases the solubility of a compound.

Key Takeaway

The formation of complex ions can substantially increase the solubility of sparingly soluble salts if the complex ion has a large K_f.

Conceptual Problems

What is the difference between K_eq and K_f?
Which would you expect to have the greater tendency to form a complex ion: Mg²⁺ or Ba²⁺? Why?
How can a ligand be used to affect the concentration of hydrated metal ions in solution? How is K_sp affected? Explain your answer.
Co(II) forms a complex ion with pyridine (C₅H₅N). Which is the Lewis acid, and which is the Lewis base? Use Lewis electron structures to justify your answer.

Numerical Problems

Fe(II) forms the complex ion [Fe(OH)₄]²⁻ through equilibrium reactions in which hydroxide replaces water in a stepwise manner. If log K₁ = 5.56, log K₂ = 4.21, log K₃ = −0.10, and log K₄ = −1.09, what is K_f? Write the equilibrium equation that corresponds to each stepwise equilibrium constant. Do you expect the [Fe(OH)₄]²⁻ complex to be stable? Explain your reasoning.
Zn(II) forms the complex ion [Zn(NH₃)₄]²⁺ through equilibrium reactions in which ammonia replaces coordinated water molecules in a stepwise manner. If log K₁ = 2.37, log K₂ = 2.44, log K₃ = 2.50, and log K₄ = 2.15, what is the overall K_f? Write the equilibrium equation that corresponds to each stepwise equilibrium constant. Do you expect the [Zn(NH₃)₄]²⁺ complex to be stable? Explain your reasoning.
Although thallium has limited commercial applications because it is toxic to humans (10 mg/kg body weight is fatal to children), it is used as a substitute for mercury in industrial switches. The complex ion [TlBr₆]³⁻ is highly stable, with log K_f = 31.6. What is the concentration of Tl(III)(aq) in equilibrium with a 1.12 M solution of Na₃[TlBr₆]?

Answer

$\begin{matrix} {{[Fe(H}_{2} {O)}_{6}]}^{2 +} (aq) + {OH}^{-} (aq) ⇌ [Fe {(H_{2} O (aq))}_{5} (OH)] + (aq) + H_{2} O & \log K_{1} = 5.56 \end{matrix}$ $\begin{matrix} [Fe {(H_{2} O)}_{5} (OH)] + (aq) + {OH}^{-} (aq) ⇌ {[Fe(H}_{2} {O)}_{4} {(OH)}_{2}](aq) + H_{2} O (aq) & \log K_{2} = 9.77 \end{matrix}$ $\begin{matrix} {[Fe(H}_{2} {O)}_{4} {(OH)}_{2}](aq) + {OH}^{-} (aq) ⇌ {{[Fe(H}_{2} {O)}_{3} {(OH)}_{3}]}^{-} (aq) + H_{2} O (aq) & \log K_{3} = 9.67 \end{matrix}$ $\begin{matrix} {{[Fe(H}_{2} {O)}_{3} {(OH)}_{3}]}^{-} (aq) + {OH}^{-} (aq) ⇌ {{[Fe(OH)}_{4}]}^{2 -} (aq) + {3H}_{2} O(l) & \log K_{4} = 8.58 \end{matrix}$ $\begin{matrix} {[Fe {(H_{2} O)}_{6}]}^{2 +} (aq) + 4 {OH}^{-} (aq) & ⇌ {[{Fe(OH)}_{4}]}^{2 -} (aq) + {6H}_{2} O(l) \\ \log K_{f} & = \log K_{1} + \log K_{2} + \log K_{2} + \log K_{4} \\ = 33.58 \end{matrix}$
Thus, K_f = 3.8 × 10³³. Because [Fe(OH)₄]²⁻ has a very large value of K_f, it should be stable in the presence of excess OH⁻.