Electrochemical Cells and Thermodynamics

19.4 Electrochemical Cells and Thermodynamics

Learning Objectives

To understand the relationship between cell potential and the equilibrium constant.
To use cell potentials to calculate solution concentrations.

Changes in reaction conditions can have a tremendous effect on the course of a redox reaction. For example, under standard conditions, the reaction of Co(s) with Ni²⁺(aq) to form Ni(s) and Co²⁺(aq) occurs spontaneously, but if we reduce the concentration of Ni²⁺ by a factor of 100, so that [Ni²⁺] is 0.01 M, then the reverse reaction occurs spontaneously instead. The relationship between voltage and concentration is one of the factors that must be understood to predict whether a reaction will be spontaneous.

The Relationship between Cell Potential and Free Energy

Electrochemical cells convert chemical energy to electrical energy and vice versa. The total amount of energy produced by an electrochemical cell, and thus the amount of energy available to do electrical work, depends on both the cell potential and the total number of electrons that are transferred from the reductant to the oxidant during the course of a reaction. The resulting electric current is measured in coulombs (C)The SI unit of measure for the number of electrons that pass a given point in 1 second; it is defined as $6.25 \times 10^{18} e^{-} /s$ and relates electron potential (in volts) to energy (in joules): 1 J/1 V = 1 C., an SI unit that measures the number of electrons passing a given point in 1 s. A coulomb relates energy (in joules) to electrical potential (in volts). Electric current is measured in amperes (A)The fundamental SI unit of electric current; it is defined as the flow of 1 C/s past a given point: 1A = 1 C/s.; 1 A is defined as the flow of 1 C/s past a given point (1 C = 1 A·s):

Equation 19.48

\frac{1 J}{1 V} = 1 C = A \cdot s

In chemical reactions, however, we need to relate the coulomb to the charge on a mole of electrons. Multiplying the charge on the electron by Avogadro’s number gives us the charge on 1 mol of electrons, which is called the faraday (F)The charge on 1 mol of electrons; it is obtained by multiplying the charge on the electron by Avogadro’s number., named after the English physicist and chemist Michael Faraday (1791–1867):

Equation 19.49

\begin{array}{l} F & = (1.60218 \times 10^{- 19} C) (\frac{6.02214 \times 10^{23}}{1 {mol e}^{-}}) \\ = 9.64855 \times 10^{4} {C/mol e}^{-} ≃ 96,486 / (V \cdot {mol e}^{-}) \end{array}

The total charge transferred from the reductant to the oxidant is therefore nF, where n is the number of moles of electrons.

Michael Faraday (1791–1867)

Faraday was a British physicist and chemist who was arguably one of the greatest experimental scientists in history. The son of a blacksmith, Faraday was self-educated and became an apprentice bookbinder at age 14 before turning to science. His experiments in electricity and magnetism made electricity a routine tool in science and led to both the electric motor and the electric generator. He discovered the phenomenon of electrolysis and laid the foundations of electrochemistry. In fact, most of the specialized terms introduced in this chapter (electrode, anode, cathode, and so forth) are due to Faraday. In addition, he discovered benzene and invented the system of oxidation state numbers that we use today. Faraday is probably best known for “The Chemical History of a Candle,” a series of public lectures on the chemistry and physics of flames.

The maximum amount of work that can be produced by an electrochemical cell (w_max) is equal to the product of the cell potential (E_cell) and the total charge transferred during the reaction (nF):

Equation 19.50

w_max = nFE_cell

Work is expressed as a negative number because work is being done by a system (an electrochemical cell with a positive potential) on its surroundings.

As you learned in Chapter 18 "Chemical Thermodynamics", the change in free energy (ΔG) is also a measure of the maximum amount of work that can be performed during a chemical process (ΔG = w_max). Consequently, there must be a relationship between the potential of an electrochemical cell and ΔG, the most important thermodynamic quantity discussed in Chapter 18 "Chemical Thermodynamics". This relationship is as follows:

Equation 19.51

ΔG = −nFE_cell

A spontaneous redox reaction is therefore characterized by a negative value of ΔG and a positive value of E_cell, consistent with our earlier discussions. When both reactants and products are in their standard states, the relationship between ΔG° and E°_cell is as follows:

Equation 19.52

ΔG° = −nFE°_cell

Note the Pattern

A spontaneous redox reaction is characterized by a negative value of ΔG°, which corresponds to a positive value of E°_cell.

Example 7

Suppose you want to prepare elemental bromine from bromide using the dichromate ion as an oxidant. Using the data in Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C", calculate the free-energy change (ΔG°) for this redox reaction under standard conditions. Is the reaction spontaneous?

Given: redox reaction

Asked for: ΔG° for the reaction and spontaneity

Strategy:

A From the relevant half-reactions and the corresponding values of E°, write the overall reaction and calculate E°_cell using Equation 19.10.

B Determine the number of electrons transferred in the overall reaction. Then use Equation 19.52 to calculate ΔG°. If ΔG° is negative, then the reaction is spontaneous.

Solution:

A As always, the first step is to write the relevant half-reactions and use them to obtain the overall reaction and the magnitude of E°. From Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C", we can find the reduction and oxidation half-reactions and corresponding E° values:

\begin{array}{l} cathode: & {Cr}_{2} O_{7}^{2 -} (aq) + 14 H^{+} (aq) + 6 e^{-} \to 2 {Cr}^{3 +} (aq) + 7 H_{2} O(l) & E_{cathode}^{°} = 1.23 V \\ anode: & {2Br}^{-} (aq) \to {Br}_{2} (aq) + 2 e^{-} & E_{anode}^{°} = 1.09 V \end{array}

To obtain the overall balanced chemical equation, we must multiply both sides of the oxidation half-reaction by 3 to obtain the same number of electrons as in the reduction half-reaction, remembering that the magnitude of E° is not affected:

\begin{array}{l} cathode: & {Cr}_{2} O_{7}^{2 -} (aq) + 14 H^{+} (aq) + 6 e^{-} \to 2 {Cr}^{3 +} (aq) + 7 H_{2} O(l) & E °_{cathode} = 1.23 V \\ anode: & {6Br}^{-} (aq) \to 3 {Br}_{2} (aq) + 6 e^{-} & E °_{anode} = 1.09 V \\ overall: & {Cr}_{2} O_{7}^{2 -} (aq) + 6 {Br}^{-} (aq) + 14 H^{+} (aq) \to & E °_{cell} = 0.14 V \\ 2 {Cr}^{3 +} (aq) + 3 {Br}_{2} (aq) + 7 H_{2} O(l) \end{array}

B We can now calculate ΔG° using Equation 19.52. Because six electrons are transferred in the overall reaction, the value of n is 6:

\begin{matrix} Δ G ° = - (n) (F) (E_{cell}^{°}) & = - (6 mole) [96,468 J/ (V \cdot mol) (0.14 V)] \\ = - 8.1 \times 10^{4} J \\ = - 81 {kJ/mol Cr}_{2} O_{7}^{2 -} \end{matrix}

Thus ΔG° is −81 kJ for the reaction as written, and the reaction is spontaneous.

Exercise

Use the data in Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C" to calculate ΔG° for the reduction of ferric ion by iodide:

2Fe³⁺(aq) + 2I⁻(aq) → 2Fe²⁺(aq) + I₂(s)

Is the reaction spontaneous?

Answer: −44 kJ/mol I₂; yes

Potentials for the Sums of Half-Reactions

Although Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C" and Chapter 29 "Appendix E: Standard Reduction Potentials at 25°C" list several half-reactions, many more are known. When the standard potential for a half-reaction is not available, we can use relationships between standard potentials and free energy to obtain the potential of any other half-reaction that can be written as the sum of two or more half-reactions whose standard potentials are available. For example, the potential for the reduction of Fe³⁺(aq) to Fe(s) is not listed in the table, but two related reductions are given:

Equation 19.53

\begin{matrix} {Fe}^{3 +} (aq) + e^{-} \to {Fe}^{2 +} (aq) & E ° = 0.77 V \end{matrix}

Equation 19.54

\begin{matrix} {Fe}^{2 +} (aq) + 2 e^{-} \to Fe(aq) & E ° = - 0.45 V \end{matrix}

Although the sum of these two half-reactions gives the desired half-reaction, we cannot simply add the potentials of two reductive half-reactions to obtain the potential of a third reductive half-reaction because E° is not a state function. However, because ΔG° is a state function, the sum of the ΔG° values for the individual reactions gives us ΔG° for the overall reaction, which is proportional to both the potential and the number of electrons (n) transferred. To obtain the value of E° for the overall half-reaction, we first must add the values of ΔG° (= −nFE°) for each individual half-reaction to obtain ΔG° for the overall half-reaction:

Equation 19.55

\begin{matrix} {Fe}^{3 +} (aq) + e^{-} & \to {Fe}^{2 +} (aq) & Δ G ° & = - (1) (F) (0.77 V) \\ {Fe}^{2 +} (aq) + 2 e^{-} & \to Fe(s) & Δ G ° & = - (2) (F) (- .045 V) \\ {Fe}^{3 +} (aq) + 3 e^{-} & \to Fe(s) & Δ G ° & = [- (1) (F) (0.77 V)] + [- (2) (F) (- 0.45 V)] \end{matrix}

Solving the last expression for ΔG° for the overall half-reaction,

Equation 19.56

ΔG° = F[(−0.77 V) + (−2)(−0.45 V)] = F(0.13 V)

Three electrons (n = 3) are transferred in the overall reaction (Equation 19.55), so substituting into Equation 19.52 and solving for E° gives the following:

\begin{matrix} Δ G ° & = - n F E_{cell}^{°} \\ F (0.13 V) & = - (3) (F) (E_{cell}^{°}) \\ E ° & = - \frac{0.13 V}{3} = - 0.043 V \end{matrix}

This value of E° is very different from the value that is obtained by simply adding the potentials for the two half-reactions (0.32 V) and even has the opposite sign.

Note the Pattern

Values of E° for half-reactions cannot be added to give E° for the sum of the half-reactions; only values of ΔG° = −nFE°_cell for half-reactions can be added.

The Relationship between Cell Potential and the Equilibrium Constant

We can use the relationship between ΔG° and the equilibrium constant K, defined in Chapter 18 "Chemical Thermodynamics", to obtain a relationship between E°_cell and K. Recall that for a general reaction of the type aA + bB → cC + dD, the standard free-energy change and the equilibrium constant are related by the following equation:

Equation 19.57

ΔG° = −RT ln K

Given the relationship between the standard free-energy change and the standard cell potential (Equation 19.52), we can write

Equation 19.58

−nFE°_cell = −RT ln K

Rearranging this equation,

Equation 19.59

E_{cell}^{°} = (\frac{R T}{n F}) ln K

For T = 298 K, Equation 19.59 can be simplified as follows:

Equation 19.60

E_{cell}^{°} = (\frac{R T}{n F}) ln K = [\frac{[8.314 J / (mol \cdot K) (298 K)]}{n [96,486 J / (V \cdot mol)]}] 2.303 log K = (\frac{0.0591 V}{n}) \log K

Thus E°_cell is directly proportional to the logarithm of the equilibrium constant. This means that large equilibrium constants correspond to large positive values of E°_cell and vice versa.

Example 8

Use the data in Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C" to calculate the equilibrium constant for the reaction of metallic lead with PbO₂ in the presence of sulfate ions to give PbSO₄ under standard conditions. (This reaction occurs when a car battery is discharged.) Report your answer to two significant figures.

Given: redox reaction

Asked for: K

Strategy:

A Write the relevant half-reactions and potentials. From these, obtain the overall reaction and E°_cell.

B Determine the number of electrons transferred in the overall reaction. Use Equation 19.60 to solve for log K and then K.

Solution:

A The relevant half-reactions and potentials from Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C" are as follows:

\begin{array}{l} cathode: & {PbO}_{2} (s) + {SO}_{4}^{2 -} (aq) + 4 H^{+} (aq) + 2 e^{-} \to {PbSO}_{4} (s) + 2 H_{2} O(l) & E_{cathode}^{°} = 1.69 V \\ anode: & Pb(s) + {SO}_{4}^{2 -} (aq) \to {PbSO}_{4} (s) + 2 e^{-} & E_{anode}^{°} = - 0.36 V \\ overall: & Pb(s) + {PbO}_{2} (s) + 2 {SO}_{4}^{2 -} (aq) + 4 H^{+} (aq) \to 2 {PbSO}_{4} (s) + 2 H_{2} O(l) & E_{cell}^{°} = 2.05 V \end{array}

B Two electrons are transferred in the overall reaction, so n = 2. Solving Equation 19.60 for log K and inserting the values of n and E°,

\begin{matrix} \log K & = \frac{n E °}{0.0591 V} = \frac{2 (2.05 V)}{0.0591 V} = 69.37 \\ K & = 2.3 \times 10^{69} \end{matrix}

Thus the equilibrium lies far to the right, favoring a discharged battery (as anyone who has ever tried unsuccessfully to start a car after letting it sit for a long time will know).

Exercise

Use the data in Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C" to calculate the equilibrium constant for the reaction of Sn²⁺(aq) with oxygen to produce Sn⁴⁺(aq) and water under standard conditions. Report your answer to two significant figures. The reaction is as follows:

2 {Sn}^{2 +} (aq) + O_{2} (g) + 4 H^{+} (aq) ⇌ 2 {Sn}^{4 +} (aq) + 2 H_{2} O(l)

Answer: 1.2 × 10⁷³

Figure 19.10 "The Relationships among Criteria for Thermodynamic Spontaneity" summarizes the relationships that we have developed based on properties of the system—that is, based on the equilibrium constant, standard free-energy change, and standard cell potential—and the criteria for spontaneity (ΔG° < 0). Unfortunately, these criteria apply only to systems in which all reactants and products are present in their standard states, a situation that is seldom encountered in the real world. A more generally useful relationship between cell potential and reactant and product concentrations, as we are about to see, uses the relationship between ΔG and the reaction quotient Q developed in Chapter 18 "Chemical Thermodynamics".

Figure 19.10 The Relationships among Criteria for Thermodynamic Spontaneity

The three properties of a system that can be used to predict the spontaneity of a redox reaction under standard conditions are K, ΔG°, and E°_cell. If we know the value of one of these quantities, then these relationships enable us to calculate the value of the other two. The signs of ΔG° and E°_cell and the magnitude of K determine the direction of spontaneous reaction under standard conditions.

The Effect of Concentration on Cell Potential: The Nernst Equation

Recall from Chapter 18 "Chemical Thermodynamics" that the actual free-energy change for a reaction under nonstandard conditions, ΔG, is given as follows:

Equation 19.61

ΔG = ΔG° + RT ln Q

We also know that ΔG = −nFE_cell and ΔG° = −nFE°_cell. Substituting these expressions into Equation 19.61, we obtain

Equation 19.62

−nFE_cell = −nFE°_cell + RT ln Q

Dividing both sides of this equation by −nF,

Equation 19.63

E_{cell} = E_{cell}^{°} - (\frac{R T}{n F}) ln Q

Equation 19.44 is called the Nernst equationAn equation for calculating cell potentials $(E_{cell})$ under nonstandard conditions; it can be used to determine the direction of spontaneous reaction for any redox reaction under an conditions: $E_{cell} = E_{cell}^{°} - (R T / n F) ln Q .$ , after the German physicist and chemist Walter Nernst (1864–1941), who first derived it. The Nernst equation is arguably the most important relationship in electrochemistry. When a redox reaction is at equilibrium (ΔG = 0), Equation 19.44 reduces to Equation 19.59 because Q = K, and there is no net transfer of electrons (i.e., E_cell = 0).

Substituting the values of the constants into Equation 19.44 with T = 298 K and converting to base-10 logarithms give the relationship of the actual cell potential (E_cell), the standard cell potential (E°_cell), and the reactant and product concentrations at room temperature (contained in Q):

Equation 19.64

E_{cell} = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log Q

Note the Pattern

The Nernst equation can be used to determine the value of E_cell, and thus the direction of spontaneous reaction, for any redox reaction under any conditions.

Equation 19.64 allows us to calculate the potential associated with any electrochemical cell at 298 K for any combination of reactant and product concentrations under any conditions. We can therefore determine the spontaneous direction of any redox reaction under any conditions, as long as we have tabulated values for the relevant standard electrode potentials. Notice in Equation 19.64 that the cell potential changes by 0.0591/n V for each 10-fold change in the value of Q because log 10 = 1.

Example 9

In the exercise in Example 6, you determined that the following reaction proceeds spontaneously under standard conditions because E°_cell > 0 (which you now know means that ΔG° < 0):

2 {Ce}^{4 +} (aq) + 2 {Cl}^{-} (aq) \to 2 {Ce}^{3 +} (aq) + {Cl}_{2} (g) E_{cell}^{°} = 0. 25 V

Calculate E for this reaction under the following nonstandard conditions and determine whether it will occur spontaneously: [Ce⁴⁺] = 0.013 M, [Ce³⁺] = 0.60 M, [Cl⁻] = 0.0030 M, $P_{{Cl}_{2}}$ = 1.0 atm, and T = 25°C.

Given: balanced redox reaction, standard cell potential, and nonstandard conditions

Asked for: cell potential

Strategy:

Determine the number of electrons transferred during the redox process. Then use the Nernst equation to find the cell potential under the nonstandard conditions.

Solution:

We can use the information given and the Nernst equation to calculate E_cell. Moreover, because the temperature is 25°C (298 K), we can use Equation 19.64 instead of 19.46. The overall reaction involves the net transfer of two electrons:

2Ce⁴⁺(aq) + 2e⁻ → 2Ce³⁺(aq) 2Cl⁻(aq) → Cl₂(g) + 2e⁻

so n = 2. Substituting the concentrations given in the problem, the partial pressure of Cl₂, and the value of E°_cell into Equation 19.64,

\begin{matrix} E_{cell} & = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log Q \\ = 0.25 V - (\frac{0.0591 V}{2}) \log (\frac{{[{Ce}^{3 +}]}^{2} P_{{Cl}_{2}}}{{[{Ce}^{4 +}]}^{2} {[{Cl}^{-}]}^{2}}) \\ = 0.25 V - [(0.0296 V) (8.37)] = 0.00 V \end{matrix}

Thus the reaction will not occur spontaneously under these conditions (because E = 0 V and ΔG = 0). The composition specified is that of an equilibrium mixture.

Exercise

In the exercise in Example 6, you determined that molecular oxygen will not oxidize MnO₂ to permanganate via the reaction

\begin{matrix} 4 {MnO}_{2} (s) + 3 O_{2} (g) + 4 {OH}^{-} (aq) \to 4 {MnO}_{4}^{-} (aq) + 2 H_{2} O(l) & E_{cell}^{°} = - 0.20 V \end{matrix}

Calculate E_cell for the reaction under the following nonstandard conditions and decide whether the reaction will occur spontaneously: pH 10, $P_{O_{2}}$ = 0.20 atm, [MNO₄⁻] = 1.0 × 10⁻⁴ M, and T = 25°C.

Answer: E_cell = −0.22 V; the reaction will not occur spontaneously.

Applying the Nernst equation to a simple electrochemical cell such as the Zn/Cu cell discussed in Section 19.2 "Standard Potentials" allows us to see how the cell voltage varies as the reaction progresses and the concentrations of the dissolved ions change. Recall that the overall reaction for this cell is as follows:

Equation 19.65

\begin{matrix} Zn(s) + {Cu}^{2 +} (aq) \to {Zn}^{2 +} (aq) + Cu(s) & E_{cell}^{°} = 1.10 V \end{matrix}

The reaction quotient is therefore Q = [Zn²⁺]/[Cu²⁺]. Suppose that the cell initially contains 1.0 M Cu²⁺ and 1.0 × 10⁻⁶ M Zn²⁺. The initial voltage measured when the cell is connected can then be calculated from Equation 19.64:

Equation 19.66

\begin{matrix} E_{cell} & = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log \frac{[{Zn}^{2 +}]}{[{Cu}^{2 +}]} \\ = 1.10 V - (\frac{0.0591 V}{2}) \log (\frac{1.0 \times 10^{- 6}}{1.0}) = 1.28 V \end{matrix}

Thus the initial voltage is greater than E° because Q < 1. As the reaction proceeds, [Zn²⁺] in the anode compartment increases as the zinc electrode dissolves, while [Cu²⁺] in the cathode compartment decreases as metallic copper is deposited on the electrode. During this process, the ratio Q = [Zn²⁺]/[Cu²⁺] steadily increases, and the cell voltage therefore steadily decreases. Eventually, [Zn²⁺] = [Cu²⁺], so Q = 1 and E_cell = E°_cell. Beyond this point, [Zn²⁺] will continue to increase in the anode compartment, and [Cu²⁺] will continue to decrease in the cathode compartment. Thus the value of Q will increase further, leading to a further decrease in E_cell. When the concentrations in the two compartments are the opposite of the initial concentrations (i.e., 1.0 M Zn²⁺ and 1.0 × 10⁻⁶ M Cu²⁺), Q = 1.0 × 10⁶, and the cell potential will be reduced to 0.92 V.

The variation of E_cell with log Q over this range is linear with a slope of −0.0591/n, as illustrated in Figure 19.11 "The Variation of ". As the reaction proceeds still further, Q continues to increase, and E_cell continues to decrease. If neither of the electrodes dissolves completely, thereby breaking the electrical circuit, the cell voltage will eventually reach zero. This is the situation that occurs when a battery is “dead.” The value of Q when E_cell = 0 is calculated as follows:

Equation 19.67

\begin{matrix} E_{cell} & = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log Q = 0 \\ E ° & = (\frac{0.0591 V}{n}) \log Q \\ \log Q & = \frac{E ° n}{0.0591 V} = \frac{(1.10 V) (2)}{0.0591 V} = 37.23 \\ Q & = 10^{37.23} = 1.7 \times 10^{37} \end{matrix}

Figure 19.11 The Variation of E_cell with Log Q for a Zn/Cu Cell

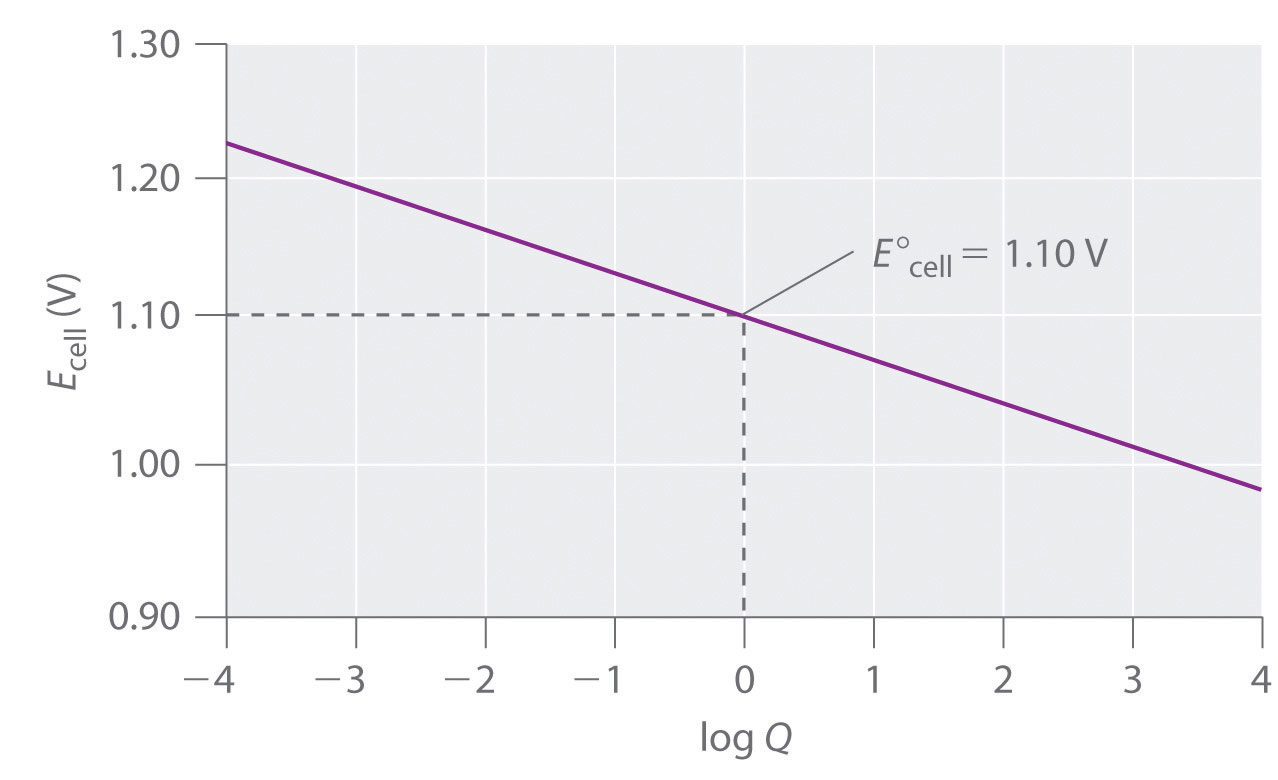
Initially, log Q < 0, and the voltage of the cell is greater than E°_cell. As the reaction progresses, log Q increases, and E_cell decreases. When [Zn²⁺] = [Cu²⁺], log Q = 0 and E_cell = E°_cell = 1.10 V. As long as the electrical circuit remains intact, the reaction will continue, and log Q will increase until Q = K and the cell voltage reaches zero. At this point, the system will have reached equilibrium.

Recall that at equilibrium, Q = K. Thus the equilibrium constant for the reaction of Zn metal with Cu²⁺ to give Cu metal and Zn²⁺ is 1.7 × 10³⁷ at 25°C.

Concentration Cells

A voltage can also be generated by constructing an electrochemical cell in which each compartment contains the same redox active solution but at different concentrations. The voltage is produced as the concentrations equilibrate. Suppose, for example, we have a cell with 0.010 M AgNO₃ in one compartment and 1.0 M AgNO₃ in the other. The cell diagram and corresponding half-reactions are as follows:

Equation 19.68

Ag(s)∣Ag⁺(aq, 0.010 M)∥Ag⁺(aq, 1.0 M)∣Ag(s)

Equation 19.69

cathode: Ag⁺(aq, 1.0 M) + e⁻ → Ag(s)

Equation 19.70

anode: Ag(s) → Ag⁺(aq, 0.010 M) + e⁻

Equation 19.71

overall: {Ag}^{+} (aq, 1 .0 M) \to {Ag}^{+} (aq, 0 .010 M)

As the reaction progresses, the concentration of Ag⁺ will increase in the left (oxidation) compartment as the silver electrode dissolves, while the Ag⁺ concentration in the right (reduction) compartment decreases as the electrode in that compartment gains mass. The total mass of Ag(s) in the cell will remain constant, however. We can calculate the potential of the cell using the Nernst equation, inserting 0 for E°_cell because E°_cathode = −E°_anode:

E_{cell} = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log Q = 0 - (\frac{0.0591 V}{1}) \log (\frac{0.010}{1.0}) = 0.12 V

An electrochemical cell of this type, in which the anode and cathode compartments are identical except for the concentration of a reactant, is called a concentration cellAn electrochemical cell in which the anode and the cathode compartments are identical except for the concentration of a reactant.. As the reaction proceeds, the difference between the concentrations of Ag⁺ in the two compartments will decrease, as will E_cell. Finally, when the concentration of Ag⁺ is the same in both compartments, equilibrium will have been reached, and the measured potential difference between the two compartments will be zero (E_cell = 0).

Example 10

Calculate the voltage in a galvanic cell that contains a manganese electrode immersed in a 2.0 M solution of MnCl₂ as the cathode, and a manganese electrode immersed in a 5.2 × 10⁻² M solution of MnSO₄ as the anode (T = 25°C).

Given: galvanic cell, identities of the electrodes, and solution concentrations

Asked for: voltage

Strategy:

A Write the overall reaction that occurs in the cell.

B Determine the number of electrons transferred. Substitute this value into the Nernst equation to calculate the voltage.

Solution:

A This is a concentration cell, in which the electrode compartments contain the same redox active substance but at different concentrations. The anions (Cl⁻ and SO₄²⁻) do not participate in the reaction, so their identity is not important. The overall reaction is as follows:

Mn²⁺(aq, 2.0 M) → Mn²⁺(aq, 5.2 × 10⁻² M)

B For the reduction of Mn²⁺(aq) to Mn(s), n = 2. We substitute this value and the given Mn²⁺ concentrations into Equation 19.64:

E_{cell} = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log Q = 0 V - (\frac{0.0591 V}{2}) \log (\frac{5.2 \times 10^{- 2}}{2.0}) = 0.047 V

Thus manganese will dissolve from the electrode in the compartment that contains the more dilute solution and will be deposited on the electrode in the compartment that contains the more concentrated solution.

Exercise

Suppose we construct a galvanic cell by placing two identical platinum electrodes in two beakers that are connected by a salt bridge. One beaker contains 1.0 M HCl, and the other a 0.010 M solution of Na₂SO₄ at pH 7.00. Both cells are in contact with the atmosphere, with $P_{O_{2}}$ = 0.20 atm. If the relevant electrochemical reaction in both compartments is the four-electron reduction of oxygen to water, O₂(g) + 4H⁺(aq) + 4e⁻ → 2H₂O(l), what will be the potential when the circuit is closed?

Answer: 0.41 V

Using Cell Potentials to Measure Solubility Products

Because voltages are relatively easy to measure accurately using a voltmeter, electrochemical methods provide a convenient way to determine the concentrations of very dilute solutions and the solubility products (K_sp) of sparingly soluble substances. As you learned in Chapter 17 "Solubility and Complexation Equilibriums", solubility products can be very small, with values of less than or equal to 10⁻³⁰. Equilibrium constants of this magnitude are virtually impossible to measure accurately by direct methods, so we must use alternative methods that are more sensitive, such as electrochemical methods.

To understand how an electrochemical cell is used to measure a solubility product, consider the cell shown in Figure 19.12 "A Galvanic Cell for Measuring the Solubility Product of AgCl", which is designed to measure the solubility product of silver chloride: K_sp = [Ag⁺][Cl⁻]. In one compartment, the cell contains a silver wire inserted into a 1.0 M solution of Ag⁺; the other compartment contains a silver wire inserted into a 1.0 M Cl⁻ solution saturated with AgCl. In this system, the Ag⁺ ion concentration in the first compartment equals K_sp. We can see this by dividing both sides of the equation for K_sp by [Cl⁻] and substituting: [Ag⁺] = K_sp/[Cl⁻] = K_sp/1.0 = K_sp. The overall cell reaction is as follows:

Ag⁺(aq, concentrated) → Ag⁺(aq, dilute)

Thus the voltage of the concentration cell due to the difference in [Ag⁺] between the two cells is as follows:

Equation 19.72

E_{cell} = 0 V - (\frac{0.0591 V}{1}) \log (\frac{{[{Ag}^{+}]}_{dilute}}{{[{Ag}^{+}]}_{concentrated}}) = - 0.0591 V log (\frac{K_{sp}}{1.0}) = - 0.0591 V log K_{sp}

Figure 19.12 A Galvanic Cell for Measuring the Solubility Product of AgCl

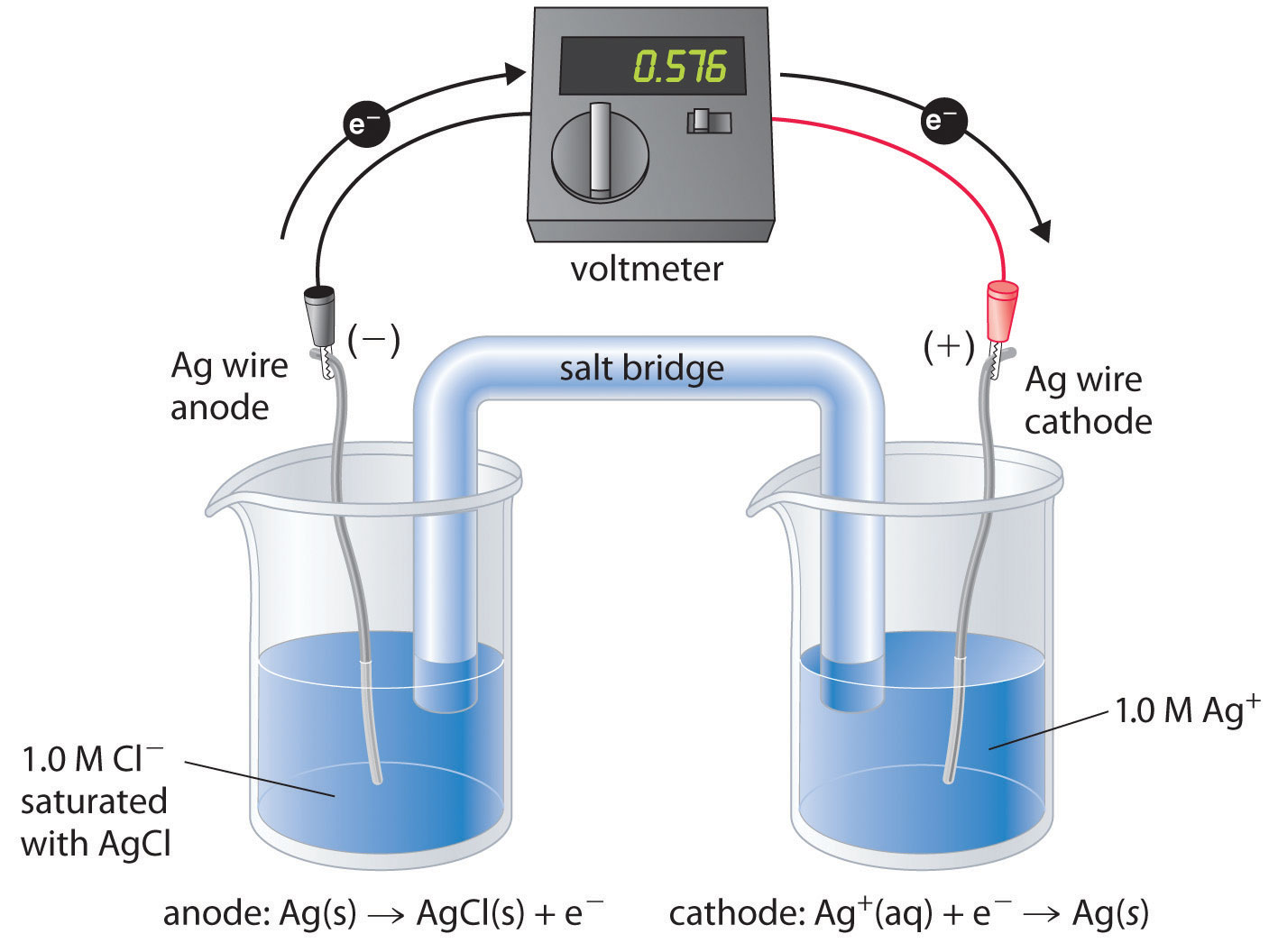
One compartment contains a silver wire inserted into a 1.0 M solution of Ag⁺, and the other compartment contains a silver wire inserted into a 1.0 M Cl⁻ solution saturated with AgCl. The potential due to the difference in [Ag⁺] between the two cells can be used to determine K_sp.

By closing the circuit, we can measure the potential caused by the difference in [Ag⁺] in the two cells. In this case, the experimentally measured voltage of the concentration cell at 25°C is 0.580 V. Solving Equation 19.72 for K_sp,

Equation 19.73

\begin{matrix} \log K_{sp} & = \frac{- E_{cell}}{0.0591 V} = \frac{- 0.580 V}{0.0591 V} = - 9.81 \\ K_{sp} & = 1.5 \times 10^{- 10} \end{matrix}

Thus a single potential measurement can provide the information we need to determine the value of the solubility product of a sparingly soluble salt.

Example 11

To measure the solubility product of lead(II) sulfate (PbSO₄) at 25°C, you construct a galvanic cell like the one shown in Figure 19.12 "A Galvanic Cell for Measuring the Solubility Product of AgCl", which contains a 1.0 M solution of a very soluble Pb²⁺ salt [lead(II) acetate trihydrate] in one compartment that is connected by a salt bridge to a 1.0 M solution of Na₂SO₄ saturated with PbSO₄ in the other. You then insert a Pb electrode into each compartment and close the circuit. Your voltmeter shows a voltage of 230 mV. What is K_sp for PbSO₄? Report your answer to two significant figures.

Given: galvanic cell, solution concentrations, electrodes, and voltage

Asked for: K _sp

Strategy:

A From the information given, write the equation for K_sp. Express this equation in terms of the concentration of Pb²⁺.

B Determine the number of electrons transferred in the electrochemical reaction. Substitute the appropriate values into Equation 19.72 and solve for K_sp.

Solution:

A You have constructed a concentration cell, with one compartment containing a 1.0 M solution of Pb²⁺ and the other containing a dilute solution of Pb²⁺ in 1.0 M Na₂SO₄. As for any concentration cell, the voltage between the two compartments can be calculated using the Nernst equation. The first step is to relate the concentration of Pb²⁺ in the dilute solution to K_sp:

\begin{matrix} [{Pb}^{2 +}] [{SO}_{4}^{2 -}] & = K_{sp} \\ [{Pb}^{2 +}] & = \frac{K_{sp}}{[{SO}_{4}^{2 -}]} = \frac{K_{sp}}{1.0 M} = K_{sp} \end{matrix}

B The reduction of Pb²⁺ to Pb is a two-electron process and proceeds according to the following reaction:

Pb²⁺(aq, concentrated) → Pb²⁺(aq, dilute)

\begin{array}{l} E_{cell} & = E_{cell}^{°} - (\frac{0.0591}{n}) \log Q \\ 0.230 V & = 0 V - (\frac{0.0591 V}{2}) \log (\frac{{[{Pb}^{2 +}]}_{dilute}}{{[{Pb}^{2 +}]}_{concentrated}}) = - 0.0296 V log (\frac{K_{sp}}{1.0}) \\ - 7.77 & = log K_{sp} \\ 1.7 \times 10^{- 8} & = K_{sp} \end{array}

Exercise

A concentration cell similar to the one described in Example 11 contains a 1.0 M solution of lanthanum nitrate [La(NO₃)₃] in one compartment and a 1.0 M solution of sodium fluoride saturated with LaF₃ in the other. A metallic La strip is inserted into each compartment, and the circuit is closed. The measured potential is 0.32 V. What is the K_sp for LaF₃? Report your answer to two significant figures.

Answer: 5.7 × 10⁻¹⁷

Using Cell Potentials to Measure Concentrations

Another use for the Nernst equation is to calculate the concentration of a species given a measured potential and the concentrations of all the other species. We saw an example of this in Example 11, in which the experimental conditions were defined in such a way that the concentration of the metal ion was equal to K_sp. Potential measurements can be used to obtain the concentrations of dissolved species under other conditions as well, which explains the widespread use of electrochemical cells in many analytical devices. Perhaps the most common application is in the determination of [H⁺] using a pH meter, as illustrated in Example 12.

Example 12

Suppose a galvanic cell is constructed with a standard Zn/Zn²⁺ couple in one compartment and a modified hydrogen electrode in the second compartment (Figure 19.7 "Determining a Standard Electrode Potential Using a Standard Hydrogen Electrode"). The pressure of hydrogen gas is 1.0 atm, but [H⁺] in the second compartment is unknown. The cell diagram is as follows:

Zn(s)∣Zn²⁺(aq, 1.0 M) ∥ H⁺(aq, ? M)∣H₂(g, 1.0 atm)∣Pt(s)

What is the pH of the solution in the second compartment if the measured potential in the cell is 0.26 V at 25°C?

Given: galvanic cell, cell diagram, and cell potential

Asked for: pH of the solution

Strategy:

A Write the overall cell reaction.

B Substitute appropriate values into the Nernst equation and solve for −log[H⁺] to obtain the pH.

Solution:

A Under standard conditions, the overall reaction that occurs is the reduction of protons by zinc to give H₂ (note that Zn lies below H₂ in Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C"):

Zn(s) + 2 H^{2 +} (aq) \to {Zn}^{2 +} (aq) + H_{2} (g) E ° = 0.76 V

B By substituting the given values into the simplified Nernst equation (Equation 19.64), we can calculate [H⁺] under nonstandard conditions:

\begin{matrix} E_{cell} & = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log (\frac{[{Zn}^{2 +}] P_{H_{2}}}{{[H^{+}]}^{2}}) \\ 0.26 V & = 0.76 V - (\frac{0.0591 V}{2}) \log (\frac{(1.0) (1.0)}{{[H^{+}]}^{2}}) \\ 16.9 & = \log (\frac{1}{{[H^{+}]}^{2}}) = \log {[H^{+}]}^{- 2} = (- 2) \log [H^{+}] \\ 8.46 & = - \log [H^{+}] \\ 8 .5 & = pH \end{matrix}

Thus the potential of a galvanic cell can be used to measure the pH of a solution.

Exercise

Suppose you work for an environmental laboratory and you want to use an electrochemical method to measure the concentration of Pb²⁺ in groundwater. You construct a galvanic cell using a standard oxygen electrode in one compartment (E°_cathode = 1.23 V). The other compartment contains a strip of lead in a sample of groundwater to which you have added sufficient acetic acid, a weak organic acid, to ensure electrical conductivity. The cell diagram is as follows”

Pb(s) ∣Pb²⁺(aq, ? M)∥H⁺(aq), 1.0 M∣O₂(g, 1.0 atm)∣Pt(s)

When the circuit is closed, the cell has a measured potential of 1.62 V. Use Table 19.3 "Comparison of Galvanic and Electrolytic Cells" and Chapter 29 "Appendix E: Standard Reduction Potentials at 25°C" to determine the concentration of Pb²⁺ in the groundwater.

Answer: 1.2 × 10⁻⁹ M

Summary

A coulomb (C) relates electrical potential, expressed in volts, and energy, expressed in joules. The current generated from a redox reaction is measured in amperes (A), where 1 A is defined as the flow of 1 C/s past a given point. The faraday (F) is Avogadro’s number multiplied by the charge on an electron and corresponds to the charge on 1 mol of electrons. The product of the cell potential and the total charge is the maximum amount of energy available to do work, which is related to the change in free energy that occurs during the chemical process. Adding together the ΔG values for the half-reactions gives ΔG for the overall reaction, which is proportional to both the potential and the number of electrons (n) transferred. Spontaneous redox reactions have a negative ΔG and therefore a positive E_cell. Because the equilibrium constant K is related to ΔG, E°_cell and K are also related. Large equilibrium constants correspond to large positive values of E°. The Nernst equation allows us to determine the spontaneous direction of any redox reaction under any reaction conditions from values of the relevant standard electrode potentials. Concentration cells consist of anode and cathode compartments that are identical except for the concentrations of the reactant. Because ΔG = 0 at equilibrium, the measured potential of a concentration cell is zero at equilibrium (the concentrations are equal). A galvanic cell can also be used to measure the solubility product of a sparingly soluble substance and calculate the concentration of a species given a measured potential and the concentrations of all the other species.

Key Takeaway

The Nernst equation can be used to determine the direction of spontaneous reaction for any redox reaction in aqueous solution.

Key Equations

Charge on a mole of electrons (faraday)

Equation 19.49: F ≈ 96,486 J/(V·mol)

Maximum work from an electrochemical cell

Equation 19.50: w_max = −nFE_cell

Relationship between Δ G ° and Δ E °

Equation 19.52: ΔG° = −nFE°_cell

Relationship between Δ G ° and K for a redox reaction

Equation 19.57: ΔG° = −RT ln K

Relationship between Δ E ° and K for a redox reaction at 25°C

Equation 19.59: $E_{cell}^{°} = (\frac{R T}{n F}) ln K$

Equation 19.60: $E_{cell}^{°} = (\frac{0.0591 V}{n}) \log K$

Relationship between Δ G ° and Q

Equation 19.61: ΔG = ΔG° + RT ln Q

Relationship between E _cell and Q at 25°C

Equation 19.64: $E_{cell}^{°} = E_{cell}^{°} - (\frac{0.0591 V}{n}) \log Q$

Conceptual Problems

State whether you agree or disagree with this reasoning and explain your answer: Standard electrode potentials arise from the number of electrons transferred. The greater the number of electrons transferred, the greater the measured potential difference. If 1 mol of a substance produces 0.76 V when 2 mol of electrons are transferred—as in Zn(s) → Zn²⁺(aq) + 2e⁻—then 0.5 mol of the substance will produce 0.76/2 V because only 1 mol of electrons is transferred.
What is the relationship between the measured cell potential and the total charge that passes through a cell? Which of these is dependent on concentration? Which is dependent on the identity of the oxidant or the reductant? Which is dependent on the number of electrons transferred?
In the equation w_max = −nFE°_cell, which quantities are extensive properties and which are intensive properties?
For any spontaneous redox reaction, E is positive. Use thermodynamic arguments to explain why this is true.
State whether you agree or disagree with this statement and explain your answer: Electrochemical methods are especially useful in determining the reversibility or irreversibility of reactions that take place in a cell.
Although the sum of two half-reactions gives another half-reaction, the sum of the potentials of the two half-reactions cannot be used to obtain the potential of the net half-reaction. Why? When does the sum of two half-reactions correspond to the overall reaction? Why?
Occasionally, you will find high-quality electronic equipment that has its electronic components plated in gold. What is the advantage of this?
Blood analyzers, which measure pH, $P_{{CO}_{2}},$ and $P_{O_{2}},$ are frequently used in clinical emergencies. For example, blood $P_{{CO}_{2}}$ is measured with a pH electrode covered with a plastic membrane that is permeable to CO₂. Based on your knowledge of how electrodes function, explain how such an electrode might work. Hint: CO₂(g) + H₂O(l) → HCO₃⁻(aq) + H⁺(aq).
Concentration cells contain the same species in solution in two different compartments. Explain what produces a voltage in a concentration cell. When does V = 0 in such a cell?
Describe how an electrochemical cell can be used to measure the solubility of a sparingly soluble salt.

Answers

extensive: w_max and n; intensive: E°_cell
Gold is highly resistant to corrosion because of its very positive reduction potential.

Numerical Problems

The chemical equation for the combustion of butane is as follows:
$C_{4} H_{10} (g) + \frac{13}{2} O_{2} (g) \to 4 {CO}_{2} (g) + 5 H_{2} O(g)$
This reaction has ΔH° = −2877 kJ/mol. Calculate E°_cell and then determine ΔG°. Is this a spontaneous process? What is the change in entropy that accompanies this process at 298 K?
How many electrons are transferred during the reaction Pb(s) + Hg₂Cl₂(s) → PbCl₂(aq) + 2Hg(l)? What is the standard cell potential? Is the oxidation of Pb by Hg₂Cl₂ spontaneous? Calculate ΔG° for this reaction.
For the cell represented as Al(s)∣Al³⁺(aq)∥Sn²⁺(aq), Sn⁴⁺(aq)∣Pt(s), how many electrons are transferred in the redox reaction? What is the standard cell potential? Is this a spontaneous process? What is ΔG°?
Explain why the sum of the potentials for the half-reactions Sn²⁺(aq) + 2e⁻ → Sn(s) and Sn⁴⁺(aq) + 2e⁻ → Sn²⁺(aq) does not equal the potential for the reaction Sn⁴⁺(aq) + 4e⁻ → Sn(s). What is the net cell potential? Compare the values of ΔG° for the sum of the potentials and the actual net cell potential.
Based on Table 19.2 "Standard Potentials for Selected Reduction Half-Reactions at 25°C" and Chapter 29 "Appendix E: Standard Reduction Potentials at 25°C", do you agree with the proposed potentials for the following half-reactions? Why or why not?
1. Cu²⁺(aq) + 2e⁻ → Cu(s), E° = 0.68 V
2. Ce⁴⁺(aq) + 4e⁻ → Ce(s), E° = −0.62 V
For each reaction, calculate E°_cell and then determine ΔG°. Indicate whether each reaction is spontaneous.
1. 2Na(s) + 2H₂O(l) → 2NaOH(aq) + H₂(g)
2. K₂S₂O₆(aq) + I₂(s) → 2KI(aq) + 2K₂SO₄(aq)
3. Sn(s) + CuSO₄(aq) → Cu(s) + SnSO₄(aq)
What is the standard change in free energy for the reaction between Ca²⁺ and Na(s) to give Ca(s) and Na⁺? Do the sign and magnitude of ΔG° agree with what you would expect based on the positions of these elements in the periodic table? Why or why not?
In acidic solution, permanganate (MnO₄⁻) oxidizes Cl⁻ to chlorine gas, and MnO₄⁻ is reduced to Mn²⁺(aq).
1. Write the balanced chemical equation for this reaction.
2. Determine E°_cell.
3. Calculate the equilibrium constant.

Potentiometric titrations are an efficient method for determining the endpoint of a redox titration. In such a titration, the potential of the solution is monitored as measured volumes of an oxidant or a reductant are added. Data for a typical titration, the potentiometric titration of Fe(II) with a 0.1 M solution of Ce(IV), are given in the following table. The starting potential has been arbitrarily set equal to zero because it is the change in potential with the addition of the oxidant that is important.

Titrant (mL)	E (mV)
2.00	50
6.00	100
9.00	255
10.00	960
11.00	1325
12.00	1625
14.00	1875

Write the balanced chemical equation for the oxidation of Fe²⁺ by Ce⁴⁺.
Plot the data and then locate the endpoint.
How many millimoles of Fe²⁺ did the solution being titrated originally contain?

The standard electrode potential (E°) for the half-reaction Ni²⁺(aq) + 2e⁻ → Ni(s) is −0.257 V. What pH is needed for this reaction to take place in the presence of 1.00 atm H₂(g) as the reductant if [Ni²⁺] is 1.00 M?
The reduction of Mn(VII) to Mn(s) by H₂(g) proceeds in five steps that can be readily followed by changes in the color of the solution. Here is the redox chemistry:
1. MnO₄⁻(aq) + e⁻ → MnO₄²⁻(aq); E° = +0.56 V (purple → dark green)
2. MnO₄²⁻(aq) + 2e⁻ + 4H⁺(aq) → MnO₂(s); E° = +2.26 V (dark green → dark brown solid)
3. MnO₂(s) + e⁻ + 4H⁺(aq) → Mn³⁺(aq); E° = +0.95 V (dark brown solid → red-violet)
4. Mn³⁺(aq) + e⁻ → Mn²⁺(aq); E° = +1.51 V (red-violet → pale pink)
5. Mn²⁺(aq) + 2e⁻ → Mn(s); E° = −1.18 V (pale pink → colorless)
1. Is the reduction of MnO₄⁻ to Mn³⁺(aq) by H₂(g) spontaneous under standard conditions? What is E°_cell?
2. Is the reduction of Mn³⁺(aq) to Mn(s) by H₂(g) spontaneous under standard conditions? What is E°_cell?
Mn(III) can disproportionate (both oxidize and reduce itself) by means of the following half-reactions:
$\begin{array}{l} {Mn}^{3 +} (aq) + e^{-} \to {Mn}^{2 +} (aq) & E ° = 1. 51 V \end{array}$ $\begin{array}{l} {Mn}^{3 +} (aq) + 2 H_{2} O(l) \to {MnO}_{2} (s) + 4 H^{+} (aq) + e^{-} & E ° = 0.95 V \end{array}$
1. What is E° for the disproportionation reaction?
2. Is disproportionation more or less thermodynamically favored at low pH than at pH 7.0? Explain your answer.
3. How could you prevent the disproportionation reaction from occurring?
For the reduction of oxygen to water, E° = 1.23 V. What is the potential for this half-reaction at pH 7.00? What is the potential in a 0.85 M solution of NaOH?
The biological molecule abbreviated as NADH (reduced nicotinamide adenine dinucleotide) can be formed by reduction of NAD⁺ (nicotinamide adenine dinucleotide) via the half-reaction NAD⁺ + H⁺ + 2e⁻ → NADH; E° = −0.32 V.
1. Would NADH be able to reduce acetate to pyruvate?
2. Would NADH be able to reduce pyruvate to lactate?
3. What potential is needed to convert acetate to lactate?
$\begin{array}{l} acetate + {CO}_{2} + {2H}^{+} + {2e}^{-} \to pyruvate + H_{2} O & E ° = - 0. 70 V \end{array}$ $\begin{array}{l} pyruvate + {2H}^{+} + {2e}^{-} \to lactate & E ° = - 0. 185 V \end{array}$
Given the following biologically relevant half-reactions, will FAD (flavin adenine dinucleotide), a molecule used to transfer electrons whose reduced form is FADH₂, be an effective oxidant for the conversion of acetaldehyde to acetate at pH 4.00?
$\begin{array}{l} acetate + {2H}^{+} + {2e}^{-} \to acetaldehyde + H_{2} O & E ° = - 0. 58 V \end{array}$ $\begin{array}{l} FAD + {2H}^{+} + {2e}^{-} \to {FADH}_{2} & E ° = - 0. 18 V \end{array}$
Ideally, any half-reaction with E° > 1.23 V will oxidize water as a result of the half-reaction O₂(g) + 4H⁺(aq) + 4e⁻ → 2H₂O(l).
1. Will FeO₄²⁻ oxidize water if the half-reaction for the reduction of Fe(VI) → Fe(III) is FeO₄²⁻(aq) + 8H⁺(aq) + 3e⁻ → Fe³⁺(aq) + 4H₂O; E° = 1.9 V?
2. What is the highest pH at which this reaction will proceed spontaneously if [Fe³⁺] = [FeO₄²⁻] = 1.0 M and $P_{O_{2}}$ = 1.0 atm?
Under acidic conditions, ideally any half-reaction with E° > 1.23 V will oxidize water via the reaction O₂(g) + 4H⁺(aq) + 4e⁻ → 2H₂O(l).
1. Will aqueous acidic KMnO₄ evolve oxygen with the formation of MnO₂?
2. At pH 14.00, what is E° for the oxidation of water by aqueous KMnO₄ (1 M) with the formation of MnO₂?
3. At pH 14.00, will water be oxidized if you are trying to form MnO₂ from MnO₄²⁻ via the reaction 2MnO₄²⁻(aq) + 2H₂O(l) → 2MnO₂(s) + O₂(g) + 4OH⁻(aq)?
Complexing agents can bind to metals and result in the net stabilization of the complexed species. What is the net thermodynamic stabilization energy that results from using CN⁻ as a complexing agent for Mn³⁺/Mn²⁺?
$\begin{array}{l} {Mn}^{3 +} (aq) + e^{-} \to {Mn}^{2 +} (aq) & E ° = 1. 51 V \end{array}$ $\begin{array}{l} {Mn(CN)}_{6}^{3 -} (aq) + e^{-} \to {Mn(CN)}_{6}^{4 -} & E ° = - 0. 24 V \end{array}$

You have constructed a cell with zinc and lead amalgam electrodes described by the cell diagram Zn(Hg)(s)∣Zn(NO₃)₂(aq)∥Pb(NO₃)₂(aq)∣Pb(Hg)(s). If you vary the concentration of Zn(NO₃)₂ and measure the potential at different concentrations, you obtain the following data:

Zn(NO₃)₂ (M)	E_cell (V)
0.0005	0.7398
0.002	0.7221
0.01	0.7014

Write the half-reactions that occur in this cell.
What is the overall redox reaction?
What is E°_cell? What is ΔG° for the overall reaction?
What is the equilibrium constant for this redox reaction?

Hydrogen gas reduces Ni²⁺ according to the following reaction: Ni²⁺(aq) + H₂(g) → Ni(s) + 2H⁺(aq); E°_cell = −0.25 V; ΔH = 54 kJ/mol.
1. What is K for this redox reaction?
2. Is this reaction likely to occur?
3. What conditions can be changed to increase the likelihood that the reaction will occur as written?
4. Is the reaction more likely to occur at higher or lower pH?
The silver–silver bromide electrode has a standard potential of 0.07133 V. What is K_sp of AgBr?

Answers

6e⁻; E°_cell = 1.813 V; the reaction is spontaneous; ΔG° = −525 kJ/mol Al.
yes; E° = 0.40 V
1. yes; E° = 0.45 V
2. 0.194 V
3. yes; E° = 0.20 V