Stoichiometry Problems

When we carry out a reaction in either an industrial setting or a laboratory, it is easier to work with masses of substances than with the numbers of molecules or moles. The general method for converting from the mass of any reactant or product to the mass of any other reactant or product using a balanced chemical equation is outlined in Figure 3.11 "A Flowchart for Stoichiometric Calculations Involving Pure Substances" and described in the following text.

Steps in Converting between Masses of Reactant and Product

1. Convert the mass of one substance (substance A) to the corresponding number of moles using its molar mass.
2. From the balanced chemical equation, obtain the number of moles of another substance (B) from the number of moles of substance A using the appropriate mole ratio (the ratio of their coefficients).
3. Convert the number of moles of substance B to mass using its molar mass. It is important to remember that some species are in excess by virtue of the reaction conditions. For example, if a substance reacts with the oxygen in air, then oxygen is in obvious (but unstated) excess.

Converting amounts of substances to moles—and vice versa—is the key to all stoichiometry problems, whether the amounts are given in units of mass (grams or kilograms), weight (pounds or tons), or volume (liters or gallons).

Figure 3.11 A Flowchart for Stoichiometric Calculations Involving Pure Substances

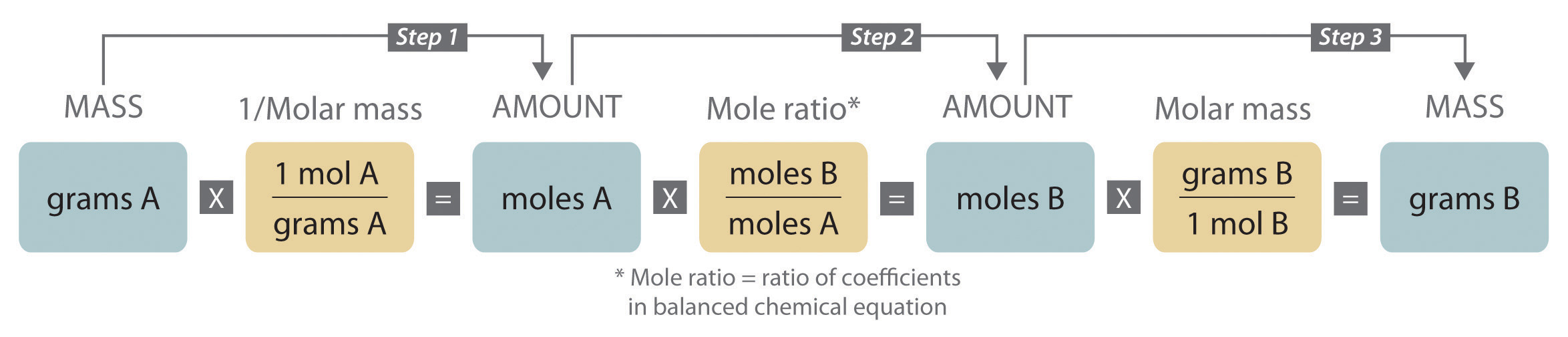
The molar masses of the reactants and the products are used as conversion factors so that you can calculate the mass of product from the mass of reactant and vice versa.

To illustrate this procedure, let’s return to the combustion of glucose. We saw earlier that glucose reacts with oxygen to produce carbon dioxide and water:

Equation 3.20

C₆H₁₂O₆(s) + 6O₂(g) → 6CO₂(g) + 6H₂O(l)

Just before a chemistry exam, suppose a friend reminds you that glucose is the major fuel used by the human brain. You therefore decide to eat a candy bar to make sure that your brain doesn’t run out of energy during the exam (even though there is no direct evidence that consumption of candy bars improves performance on chemistry exams). If a typical 2 oz candy bar contains the equivalent of 45.3 g of glucose and the glucose is completely converted to carbon dioxide during the exam, how many grams of carbon dioxide will you produce and exhale into the exam room?

The initial step in solving a problem of this type must be to write the balanced chemical equation for the reaction. Inspection of Equation 3.20 shows that it is balanced as written, so we can proceed to the strategy outlined in Figure 3.11 "A Flowchart for Stoichiometric Calculations Involving Pure Substances", adapting it as follows:

1. Use the molar mass of glucose (to one decimal place, 180.2 g/mol) to determine the number of moles of glucose in the candy bar:

   $$\text{moles glucose }=\text{ 45}\text{.3 }\cancel{\text{g glucose}}\times \frac{\text{1 mol glucose}}{\text{180}\text{.2 }\cancel{\text{g glucose}}}=\text{ 0}\text{.251 mol glucose}$$

2. According to the balanced chemical equation, 6 mol of CO₂ is produced per mole of glucose; the mole ratio of CO₂ to glucose is therefore 6:1. The number of moles of CO₂ produced is thus

   $$\begin{array}{ll}{\text{moles CO}}_{\text{2}}\hfill & =\text{ mol glucose}\times \frac{{\text{6 mol CO}}_{\text{2}}}{\text{1 mol glucose}}\hfill \\ \hfill & =\text{ 0}\text{.251 }\cancel{\text{mol glucose}}\times \frac{{\text{6 mol CO}}_{\text{2}}}{\text{1 }\cancel{\text{mol glucose}}}\hfill \\ \hfill & =\text{ 1}{\text{.51 mol CO}}_{\text{2}}\hfill \end{array}$$

3. Use the molar mass of CO₂ (44.010 g/mol) to calculate the mass of CO₂ corresponding to 1.51 mol of CO₂:

   $${\text{mass of CO}}_{\text{2}}=\text{ 1}\text{.51 }\cancel{{\text{mol CO}}_{\text{2}}}\times \frac{\text{44}{\text{.010 g CO}}_{\text{2}}}{\text{1 }\cancel{{\text{mol CO}}_{\text{2}}}}=\text{ 66}{\text{.5 g CO}}_{\text{2}}$$

We can summarize these operations as follows:

$$\text{45}\text{.3 g glucose}\times \underset{\text{step 1}}{\frac{\text{1 mol glucose}}{\text{180}\text{.2 g glucose}}}\times \underset{\text{step 2}}{\frac{{\text{6 mol CO}}_{\text{2}}}{\text{1 mol glucose}}}\times \underset{\text{step 3}}{\frac{\text{44}{\text{.010 g CO}}_{\text{2}}}{{\text{1 mol CO}}_{\text{2}}}}=\text{ 66}{\text{.4 g CO}}_{\text{2}}$$

Discrepancies between the two values are attributed to rounding errors resulting from using stepwise calculations in steps 1–3. (For more information about rounding and significant digits, see Essential Skills 1 in Chapter 1 "Introduction to Chemistry", Section 1.9 "Essential Skills 1".) In Chapter 10 "Gases", you will discover that this amount of gaseous carbon dioxide occupies an enormous volume—more than 33 L. We could use similar methods to calculate the amount of oxygen consumed or the amount of water produced.

We just used the balanced chemical equation to calculate the mass of product that is formed from a certain amount of reactant. We can also use the balanced chemical equation to determine the masses of reactants that are necessary to form a certain amount of product or, as shown in Example 11, the mass of one reactant that is required to consume a given mass of another reactant.

Example 11

The combustion of hydrogen with oxygen to produce gaseous water is extremely vigorous, producing one of the hottest flames known. Because so much energy is released for a given mass of hydrogen or oxygen, this reaction was used to fuel the NASA (National Aeronautics and Space Administration) space shuttles, which have recently been retired from service. NASA engineers calculated the exact amount of each reactant needed for the flight to make sure that the shuttles did not carry excess fuel into orbit. Calculate how many tons of hydrogen a space shuttle needed to carry for each 1.00 tn of oxygen (1 tn = 2000 lb).

The US space shuttle Discovery during liftoff. The large cylinder in the middle contains the oxygen and hydrogen that fueled the shuttle’s main engine.

Given: reactants, products, and mass of one reactant

Asked for: mass of other reactant

Strategy:

A Write the balanced chemical equation for the reaction.

B Convert mass of oxygen to moles. From the mole ratio in the balanced chemical equation, determine the number of moles of hydrogen required. Then convert the moles of hydrogen to the equivalent mass in tons.

Solution:

We use the same general strategy for solving stoichiometric calculations as in the preceding example. Because the amount of oxygen is given in tons rather than grams, however, we also need to convert tons to units of mass in grams. Another conversion is needed at the end to report the final answer in tons.

A We first use the information given to write a balanced chemical equation. Because we know the identity of both the reactants and the product, we can write the reaction as follows:

H₂(g) + O₂(g) → H₂O(g)

This equation is not balanced because there are two oxygen atoms on the left side and only one on the right. Assigning a coefficient of 2 to both H₂O and H₂ gives the balanced chemical equation:

2H₂(g) + O₂(g) → 2H₂O(g)

Thus 2 mol of H₂ react with 1 mol of O₂ to produce 2 mol of H₂O.

1. B To convert tons of oxygen to units of mass in grams, we multiply by the appropriate conversion factors:

   $${\text{mass of O}}_{\text{2}}=\text{ 1}\text{.00 }\cancel{\text{tn}}\times \frac{\text{2000 }\cancel{\text{lb}}}{\cancel{\text{tn}}}\times \frac{453.6\text{ g}}{\cancel{\text{lb}}}=9.07\times {10}^5{\text{ g O}}_{\text{2}}$$

   Using the molar mass of O₂ (32.00 g/mol, to four significant figures), we can calculate the number of moles of O₂ contained in this mass of O₂:

   $${\text{mol O}}_{\text{2}}=9.07\times {10}^5\cancel{{\text{g O}}_{\text{2}}}\times \frac{{\text{1 mol O}}_{\text{2}}}{\text{32}\text{.00 }\cancel{{\text{g O}}_{\text{2}}}}=\text{ 2}\text{.83}\times {\text{10}}^{\text{4}}{\text{ mol O}}_{\text{2}}$$

2. Now use the coefficients in the balanced chemical equation to obtain the number of moles of H₂ needed to react with this number of moles of O₂:

   $$\begin{array}{l}{\text{mol H}}_{\text{2}}={\text{mol O}}_{\text{2}}\times \frac{{\text{2 mol H}}_{\text{2}}}{{\text{1 mol O}}_{\text{2}}}\\ =\text{ 2}\text{.83}\times {\text{10}}^{\text{4}}\cancel{{\text{mol O}}_{\text{2}}}\times \frac{{\text{2 mol H}}_{\text{2}}}{\text{1 }\cancel{{\text{mol O}}_{\text{2}}}}=5.66\times {10}^4{\text{ mol H}}_{\text{2}}\end{array}$$

3. The molar mass of H₂ (2.016 g/mol) allows us to calculate the corresponding mass of H₂:

   $${\text{mass of H}}_{\text{2}}=\text{ 5}\text{.66}\times {\text{10}}^{\text{4}}\cancel{{\text{mol H}}_{\text{2}}}\times \frac{\text{2}{\text{.016 g H}}_{\text{2}}}{\cancel{{\text{mol H}}_{\text{2}}}}=1.14\times {10}^5{\text{ g H}}_{\text{2}}$$

   Finally, convert the mass of H₂ to the desired units (tons) by using the appropriate conversion factors:

   $${\text{tons H}}_{\text{2}}=\text{ 1}\text{.14}\times {\text{10}}^{\text{5}}\cancel{\text{g}}{\text{ H}}_{\text{2}}\times \frac{\text{1}\cancel{\text{lb}}}{\text{453}\text{.6 }\cancel{\text{g}}}\times \frac{\text{1 tn}}{\text{2000 }\cancel{\text{lb}}}=0.126{\text{ tn H}}_{\text{2}}$$

   The space shuttle had to be designed to carry 0.126 tn of H₂ for each 1.00 tn of O₂. Even though 2 mol of H₂ are needed to react with each mole of O₂, the molar mass of H₂ is so much smaller than that of O₂ that only a relatively small mass of H₂ is needed compared to the mass of O₂.

Exercise

Alchemists produced elemental mercury by roasting the mercury-containing ore cinnabar (HgS) in air:

HgS(s) + O₂(g) → Hg(l) + SO₂(g)

The volatility and toxicity of mercury make this a hazardous procedure, which likely shortened the life span of many alchemists. Given 100 g of cinnabar, how much elemental mercury can be produced from this reaction?

Answer: 86.2 g

Limiting Reactants

In all the examples discussed thus far, the reactants were assumed to be present in stoichiometric quantities. Consequently, none of the reactants was left over at the end of the reaction. This is often desirable, as in the case of a space shuttle, where excess oxygen or hydrogen was not only extra freight to be hauled into orbit but also an explosion hazard. More often, however, reactants are present in mole ratios that are not the same as the ratio of the coefficients in the balanced chemical equation. As a result, one or more of them will not be used up completely but will be left over when the reaction is completed. In this situation, the amount of product that can be obtained is limited by the amount of only one of the reactants. The reactant that restricts the amount of product obtained is called the limiting reactantThe reactant that restricts the amount of product obtained in a chemical reaction.. The reactant that remains after a reaction has gone to completion is in excess.

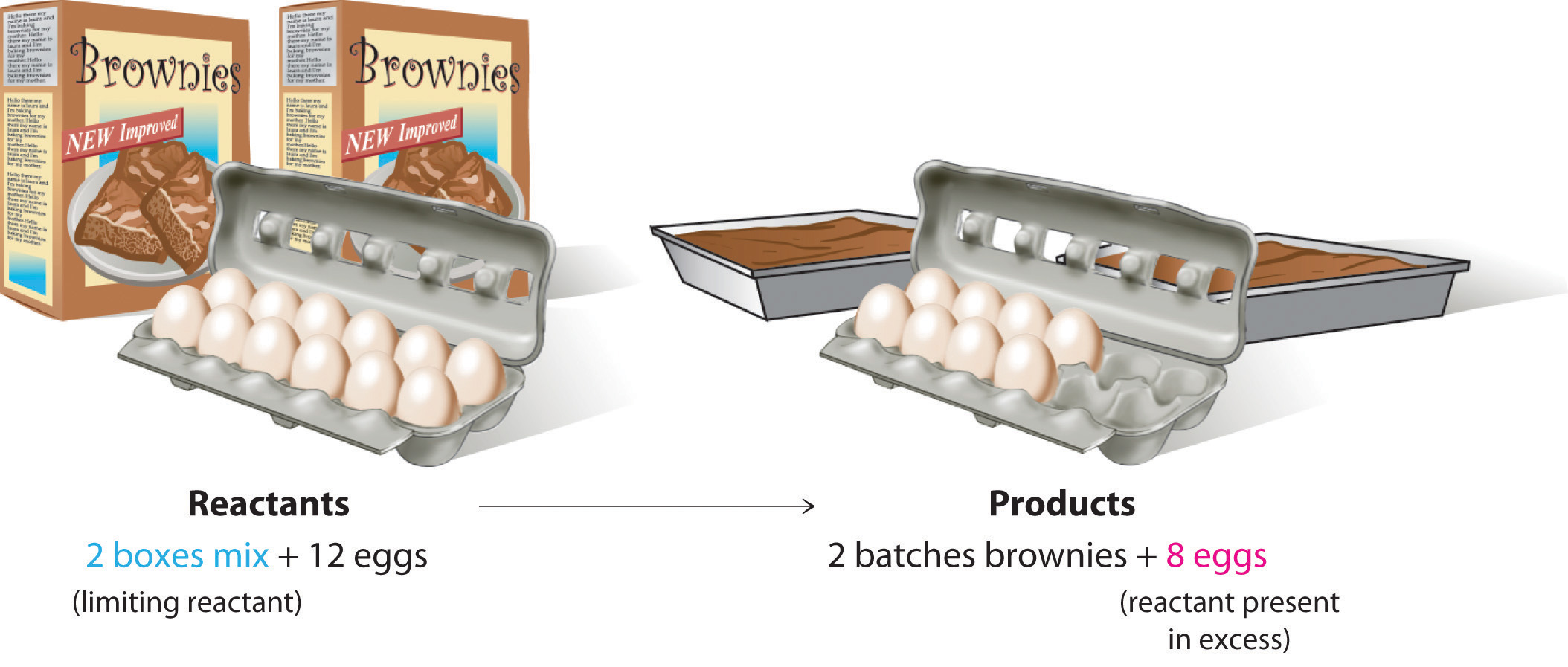
To be certain you understand these concepts, let’s first consider a nonchemical example. Assume you have invited some friends for dinner and want to bake brownies for dessert. You find two boxes of brownie mix in your pantry and see that each package requires two eggs. The balanced equation for brownie preparation is thus

If you have a dozen eggs, which ingredient will determine the number of batches of brownies that you can prepare? Because each box of brownie mix requires two eggs and you have two boxes, you need four eggs. Twelve eggs is eight more eggs than you need. Although the ratio of eggs to boxes in Equation 3.21 is 2:1, the ratio in your possession is 6:1. Hence the eggs are the ingredient (reactant) present in excess, and the brownie mix is the limiting reactant (Figure 3.12 "The Concept of a Limiting Reactant in the Preparation of Brownies"). Even if you had a refrigerator full of eggs, you could make only two batches of brownies.

Figure 3.12 The Concept of a Limiting Reactant in the Preparation of Brownies

Let’s now turn to a chemical example of a limiting reactant: the production of pure titanium. This metal is fairly light (45% lighter than steel and only 60% heavier than aluminum) and has great mechanical strength (as strong as steel and twice as strong as aluminum). Because it is also highly resistant to corrosion and can withstand extreme temperatures, titanium has many applications in the aerospace industry. Titanium is also used in medical implants and portable computer housings because it is light and resistant to corrosion. Although titanium is the ninth most common element in Earth’s crust, it is relatively difficult to extract from its ores. In the first step of the extraction process, titanium-containing oxide minerals react with solid carbon and chlorine gas to form titanium tetrachloride (TiCl₄) and carbon dioxide. Titanium tetrachloride is then converted to metallic titanium by reaction with magnesium metal at high temperature:

Because titanium ores, carbon, and chlorine are all rather inexpensive, the high price of titanium (about $100 per kilogram) is largely due to the high cost of magnesium metal. Under these circumstances, magnesium metal is the limiting reactant in the production of metallic titanium.

Medical use of titanium. Here is an example of its successful use in joint replacement implants.

Suppose you have 1.00 kg of titanium tetrachloride and 200 g of magnesium metal. How much titanium metal can you produce according to Equation 3.22? Solving this type of problem requires that you carry out the following steps:

1. Determine the number of moles of each reactant.
2. Compare the mole ratio of the reactants with the ratio in the balanced chemical equation to determine which reactant is limiting.
3. Calculate the number of moles of product that can be obtained from the limiting reactant.
4. Convert the number of moles of product to mass of product.

1. To determine the number of moles of reactants present, you must calculate or look up their molar masses: 189.679 g/mol for titanium tetrachloride and 24.305 g/mol for magnesium. The number of moles of each is calculated as follows:

   $$\begin{array}{ll}{\text{moles TiCl}}_{\text{4}}\hfill & =\frac{{\text{mass TiCl}}_{\text{4}}}{{\text{molar mass TiCl}}_{\text{4}}}\hfill \\ \hfill & =\text{ 1000 }\cancel{{\text{g TiCl}}_{\text{4}}}\times \frac{{\text{1 mol TiCl}}_{\text{4}}}{\text{189}\text{.679 }\cancel{{\text{g TiCl}}_{\text{4}}}}=\text{ 5}{\text{.272 mol TiCl}}_{\text{4}}\hfill \\ \text{moles Mg }\hfill & =\frac{\text{mass Mg}}{\text{molar mass Mg}}\hfill \\ \hfill & =\text{ 200 }\cancel{\text{g Mg}}\times \frac{\text{1 mol Mg}}{\text{24}\text{.305 }\cancel{\text{g Mg}}}=\text{ 8}\text{.23 mol Mg}\hfill \end{array}$$

2. You have more moles of magnesium than of titanium tetrachloride, but the ratio is only

   $$\frac{\text{mol Mg}}{{\text{mol TiCl}}_{\text{4}}}=\frac{\text{8}\text{.23 mol}}{\text{5}\text{.272 mol}}=\text{ 1}\text{.56}$$

   Because the ratio of the coefficients in the balanced chemical equation is

   $$\frac{\text{2 mol Mg}}{{\text{1 mol TiCl}}_{\text{4}}}=\text{ 2}$$

   you do not have enough magnesium to react with all the titanium tetrachloride. If this point is not clear from the mole ratio, you should calculate the number of moles of one reactant that is required for complete reaction of the other reactant. For example, you have 8.23 mol of Mg, so you need (8.23 ÷ 2) = 4.12 mol of TiCl₄ for complete reaction. Because you have 5.272 mol of TiCl₄, titanium tetrachloride is present in excess. Conversely, 5.272 mol of TiCl₄ requires 2 × 5.272 = 10.54 mol of Mg, but you have only 8.23 mol. So magnesium is the limiting reactant.

3. Because magnesium is the limiting reactant, the number of moles of magnesium determines the number of moles of titanium that can be formed:

   $$\text{moles Ti }=\text{ 8}\text{.23 }\cancel{\text{mol Mg}}=\frac{\text{1 mol Ti}}{\text{2 }\cancel{\text{mol Mg}}}=\text{ 4}\text{.12 mol Ti}$$

   Thus only 4.12 mol of Ti can be formed.

4. To calculate the mass of titanium metal that you can obtain, multiply the number of moles of titanium by the molar mass of titanium (47.867 g/mol):

   $$\text{moles Ti }=\text{ mass Ti }\times \text{ molar mass Ti }=\text{ 4}\text{.12 }\cancel{\text{mol Ti}}\times \frac{\text{47}\text{.867 g Ti}}{\text{1 }\cancel{\text{mol Ti}}}=\text{ 197 g Ti}$$

Here is a simple and reliable way to identify the limiting reactant in any problem of this sort:

1. Calculate the number of moles of each reactant present: 5.272 mol of TiCl₄ and 8.23 mol of Mg.

2. Divide the actual number of moles of each reactant by its stoichiometric coefficient in the balanced chemical equation:

   $$\begin{array}{rr}\hfill {\text{TiCl}}_{\text{4}}\text{: }\frac{\text{5}\text{.272 mol (actual)}}{\text{1 mol (stoich)}}=\text{ 5}\text{.272}& \hfill \text{Mg: }\frac{\text{8}\text{.23 mol (actual)}}{\text{2 mol (stoich)}}=\text{ 4}\text{.12}\end{array}$$
3. The reactant with the smallest mole ratio is limiting. Magnesium, with a calculated stoichiometric mole ratio of 4.12, is the limiting reactant.

As you learned in Chapter 1 "Introduction to Chemistry", density is the mass per unit volume of a substance. If we are given the density of a substance, we can use it in stoichiometric calculations involving liquid reactants and/or products, as Example 12 demonstrates.

Example 12

Ethyl acetate (CH₃CO₂C₂H₅) is the solvent in many fingernail polish removers and is used to decaffeinate coffee beans and tea leaves. It is prepared by reacting ethanol (C₂H₅OH) with acetic acid (CH₃CO₂H); the other product is water. A small amount of sulfuric acid is used to accelerate the reaction, but the sulfuric acid is not consumed and does not appear in the balanced chemical equation. Given 10.0 mL each of acetic acid and ethanol, how many grams of ethyl acetate can be prepared from this reaction? The densities of acetic acid and ethanol are 1.0492 g/mL and 0.7893 g/mL, respectively.

Given: reactants, products, and volumes and densities of reactants

Asked for: mass of product

Strategy:

A Balance the chemical equation for the reaction.

B Use the given densities to convert from volume to mass. Then use each molar mass to convert from mass to moles.

C Using mole ratios, determine which substance is the limiting reactant. After identifying the limiting reactant, use mole ratios based on the number of moles of limiting reactant to determine the number of moles of product.

D Convert from moles of product to mass of product.

Solution:

A We always begin by writing the balanced chemical equation for the reaction:

C₂H₅OH(l) + CH₃CO₂H(aq) → CH₃CO₂C₂H₅(aq) + H₂O(l)

B We need to calculate the number of moles of ethanol and acetic acid that are present in 10.0 mL of each. Recall from Chapter 1 "Introduction to Chemistry" that the density of a substance is the mass divided by the volume:

$$\text{density }=\frac{\text{mass}}{\text{volume}}$$

Rearranging this expression gives mass = (density)(volume). We can replace mass by the product of the density and the volume to calculate the number of moles of each substance in 10.0 mL (remember, 1 mL = 1 cm³):

$$\begin{array}{ll}{\text{moles C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH }\hfill & =\frac{{\text{mass C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}{{\text{molar mass C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}\hfill \\ \hfill & =\frac{{\text{volume C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}\times {\text{density C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}{{\text{molar mass C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}\hfill \\ \hfill & =\text{ 10}\text{.0 }\cancel{{\text{mL C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}\times \frac{\text{0}\text{.7893 }\cancel{{\text{g C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}}{\text{1 }\cancel{{\text{mL C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}}\times \frac{1{\text{ mol C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}{\text{46}\text{.07 }\cancel{{\text{g C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}}\hfill \\ \hfill & =\text{ 0}{\text{.171 mol C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}\hfill \\ {\text{moles CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H }\hfill & =\frac{{\text{mass CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}{{\text{molar mass CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}\hfill \\ \hfill & =\frac{{\text{volume CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}\times {\text{density CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}{{\text{molar mass CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}\hfill \\ \hfill & =\text{ 10}\text{.0 }\cancel{{\text{mL CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}\times \frac{\text{1}\text{.0492 }\cancel{{\text{g CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}}{\text{1 }\cancel{{\text{mL CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}}\times \frac{1{\text{ mol CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}{\text{60}\text{.05 }\cancel{{\text{g CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}}}\hfill \\ \hfill & =\text{ 0}{\text{.175 mol CH}}_{\text{3}}{\text{CO}}_{\text{2}}\text{H}\hfill \end{array}$$

C The number of moles of acetic acid exceeds the number of moles of ethanol. Because the reactants both have coefficients of 1 in the balanced chemical equation, the mole ratio is 1:1. We have 0.171 mol of ethanol and 0.175 mol of acetic acid, so ethanol is the limiting reactant and acetic acid is in excess. The coefficient in the balanced chemical equation for the product (ethyl acetate) is also 1, so the mole ratio of ethanol and ethyl acetate is also 1:1. This means that given 0.171 mol of ethanol, the amount of ethyl acetate produced must also be 0.171 mol:

$$\begin{array}{ll}\text{moles ethyl acetate }\hfill & =\text{ mol ethanol}\times \frac{\text{1 mol ethyl acetate}}{\text{1 mol ethanol}}\hfill \\ \hfill & =\text{ 0}\text{.171 }\cancel{{\text{mol C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}\times \frac{{\text{1 mol CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}}{\text{1 }\cancel{{\text{mol C}}_{\text{2}}{\text{H}}_{\text{5}}\text{OH}}}\hfill \\ \hfill & =\text{ 0}{\text{.171 mol CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}\hfill \end{array}$$

D The final step is to determine the mass of ethyl acetate that can be formed, which we do by multiplying the number of moles by the molar mass:

$$\begin{array}{ll}\text{mass of ethyl acetate }\hfill & =\text{ mol ethyl acetate }\times \text{ molar mass ethyl acetate}\hfill \\ \hfill & =\text{ 0}\text{.171 }\cancel{{\text{mol CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}}\times \frac{\text{88}{\text{.11 g CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}}{\text{1 }\cancel{{\text{mol CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}}}\hfill \\ \hfill & =\text{ 15}{\text{.1 g CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}\hfill \end{array}$$

Thus 15.1 g of ethyl acetate can be prepared in this reaction. If necessary, you could use the density of ethyl acetate (0.9003 g/cm³) to determine the volume of ethyl acetate that could be produced:

$$\begin{array}{ll}\text{volume of ethyl acetate }\hfill & =\text{ 15}\text{.1 }\cancel{{\text{g CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}}\times \frac{{\text{1 mL CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}}{\text{0}\text{.9003 }\cancel{{\text{g CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}}}\hfill \\ \hfill & =\text{ 16}{\text{.8 mL CH}}_{\text{3}}{\text{CO}}_{\text{2}}{\text{C}}_{\text{2}}{\text{H}}_{\text{5}}\hfill \end{array}$$

Exercise

Under appropriate conditions, the reaction of elemental phosphorus and elemental sulfur produces the compound P₄S₁₀. How much P₄S₁₀ can be prepared starting with 10.0 g of P₄ and 30.0 g of S₈?

Answer: 35.9 g

Percent Yield

You have learned that when reactants are not present in stoichiometric quantities, the limiting reactant determines the maximum amount of product that can be formed from the reactants. The amount of product calculated in this way is the theoretical yieldThe maximum amount of product that can be formed from the reactants in a chemical reaction, which theoretically is the amount of product that would be obtained if the reaction occurred perfectly and the method of purifying the product were 100% efficient., the amount you would obtain if the reaction occurred perfectly and your method of purifying the product were 100% efficient.

In reality, you almost always obtain less product than is theoretically possible because of mechanical losses (such as spilling), separation procedures that are not 100% efficient, competing reactions that form undesired products, and reactions that simply do not go all the way to completion, thus resulting in a mixture of products and reactants. This last possibility is a common occurrence and is the subject of Chapter 15 "Chemical Equilibrium". So the actual yieldThe measured mass of products actually obtained from a reaction. The actual yield is nearly always less than the theoretical yield., the measured mass of products obtained from a reaction, is almost always less than the theoretical yield (often much less). The percent yieldThe ratio of the actual yield of a reaction to the theoretical yield multiplied by 100 to give a percentage. of a reaction is the ratio of the actual yield to the theoretical yield, multiplied by 100 to give a percentage:

The method used to calculate the percent yield of a reaction is illustrated in Example 13.

Example 13

Procaine is a key component of Novocain, an injectable local anesthetic used in dental work and minor surgery. Procaine can be prepared in the presence of H₂SO₄ (indicated above the arrow) by the reaction

$$\underset{p\text{-aminobenzoic acid}}{{\text{C}}_{\text{7}}{\text{H}}_{\text{7}}{\text{NO}}_{\text{2}}}+\underset{\text{2-diethylaminoethanol}}{{\text{C}}_{\text{6}}{\text{H}}_{\text{15}}\text{NO}}\stackrel{{\text{H}}_{\text{2}}{\text{SO}}_4}{\to }\underset{\text{procaine}}{{\text{C}}_{\text{13}}{\text{H}}_{\text{20}}{\text{N}}_{\text{2}}{\text{O}}_{\text{2}}}+{\text{H}}_{\text{2}}\text{O}$$

If we carried out this reaction using 10.0 g of p-aminobenzoic acid and 10.0 g of 2-diethylaminoethanol, and we isolated 15.7 g of procaine, what was the percent yield?

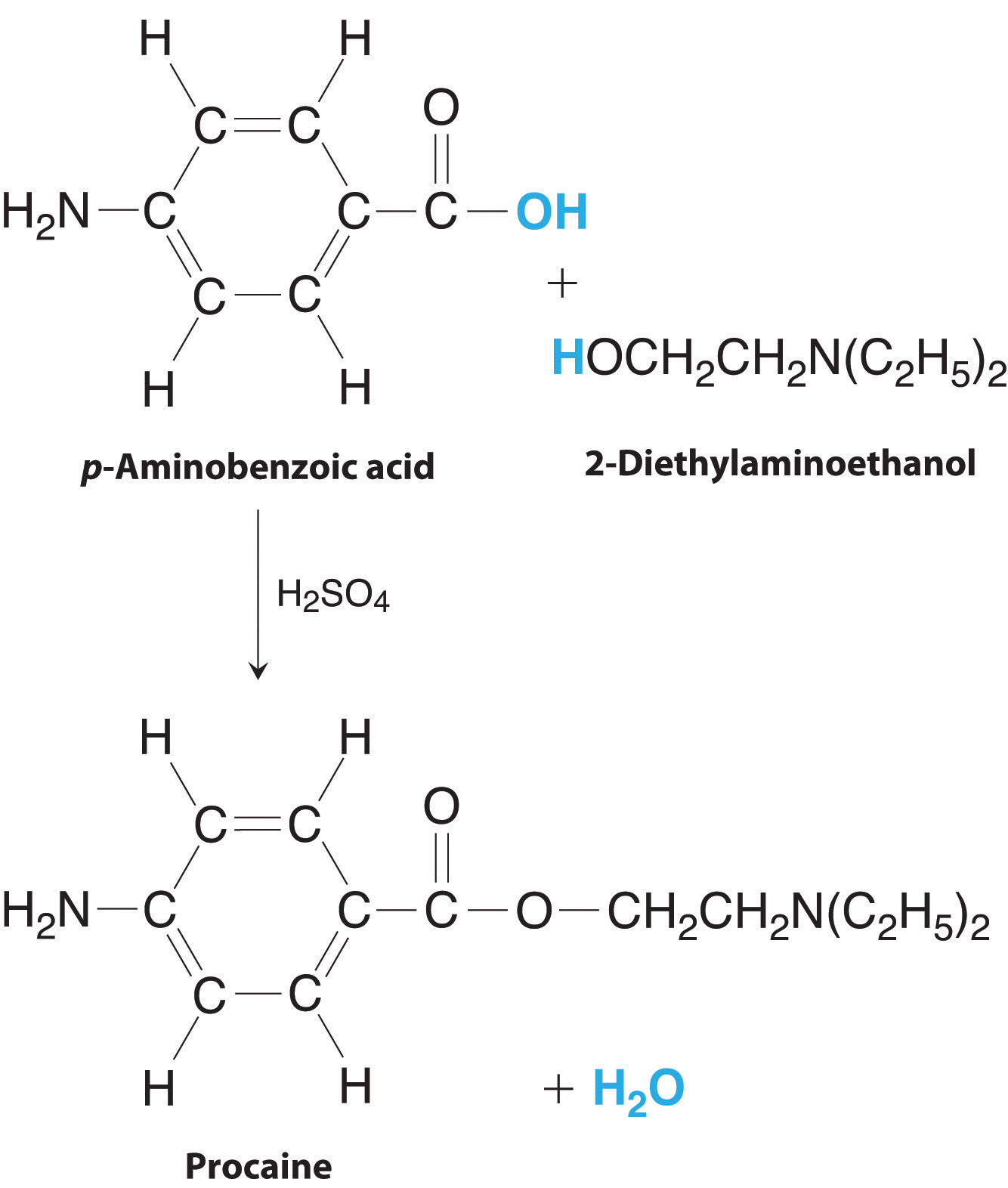
The preparation of procaine. A reaction of p-aminobenzoic acid with 2-diethylaminoethanol yields procaine and water.

Given: masses of reactants and product

Asked for: percent yield

Strategy:

A Write the balanced chemical equation.

B Convert from mass of reactants and product to moles using molar masses and then use mole ratios to determine which is the limiting reactant. Based on the number of moles of the limiting reactant, use mole ratios to determine the theoretical yield.

C Calculate the percent yield by dividing the actual yield by the theoretical yield and multiplying by 100.

Solution:

A From the formulas given for the reactants and the products, we see that the chemical equation is balanced as written. According to the equation, 1 mol of each reactant combines to give 1 mol of product plus 1 mol of water.

B To determine which reactant is limiting, we need to know their molar masses, which are calculated from their structural formulas: p-aminobenzoic acid (C₇H₇NO₂), 137.14 g/mol; 2-diethylaminoethanol (C₆H₁₅NO), 117.19 g/mol. Thus the reaction used the following numbers of moles of reactants:

$$\begin{array}{l}\text{moles }p\text{-aminobenzoic acid }=\text{ 10}\text{.0 }\cancel{\text{g}}\times \frac{\text{1 mol}}{\text{137}\text{.14 }\cancel{\text{g}}}=\text{ 0}\text{.0729 mol }p\text{-aminobenzoic acid}\\ \text{moles 2-diethylaminoethanol }=\text{ 10}\text{.0 }\cancel{\text{g}}\times \frac{\text{1 mol}}{\text{117}\text{.19 }\cancel{\text{g}}}=\text{ 0}\text{.0853 mol 2-diethylaminoethanol}\end{array}$$

The reaction requires a 1:1 mole ratio of the two reactants, so p-aminobenzoic acid is the limiting reactant. Based on the coefficients in the balanced chemical equation, 1 mol of p-aminobenzoic acid yields 1 mol of procaine. We can therefore obtain only a maximum of 0.0729 mol of procaine. To calculate the corresponding mass of procaine, we use its structural formula (C₁₃H₂₀N₂O₂) to calculate its molar mass, which is 236.31 g/mol.

$$\text{theoretical yield of procaine }=\text{ 0}\text{.0729 }\cancel{\text{mol}}\times \frac{\text{236}\text{.31 g}}{\text{1 }\cancel{\text{mol}}}=\text{ 17}\text{.2 g}$$

C The actual yield was only 15.7 g of procaine, so the percent yield was

$$\text{percent yield }=\frac{\text{15}\text{.7 g}}{\text{17}\text{.2 g}}\times 100=91.3\%$$

(If the product were pure and dry, this yield would indicate that we have very good lab technique!)

Exercise

Lead was one of the earliest metals to be isolated in pure form. It occurs as concentrated deposits of a distinctive ore called galena (PbS), which is easily converted to lead oxide (PbO) in 100% yield by roasting in air via the following reaction:

2PbS(s) + 3O₂(g) → 2PbO(s) + 2SO₂(g)

The resulting PbO is then converted to the pure metal by reaction with charcoal. Because lead has such a low melting point (327°C), it runs out of the ore-charcoal mixture as a liquid that is easily collected. The reaction for the conversion of lead oxide to pure lead is as follows:

PbO(s) + C(s) → Pb(l) + CO(g)

If 93.3 kg of PbO is heated with excess charcoal and 77.3 kg of pure lead is obtained, what is the percent yield?

Crystalline galena (a) and a sample of lead (b). Pure lead is soft enough to be shaped easily with a hammer, unlike the brittle mineral galena, the main ore of lead.

Answer: 89.2%

Percent yield can range from 0% to 100%.In the laboratory, a student will occasionally obtain a yield that appears to be greater than 100%. This usually happens when the product is impure or is wet with a solvent such as water. If this is not the case, then the student must have made an error in weighing either the reactants or the products. The law of conservation of mass applies even to undergraduate chemistry laboratory experiments! A 100% yield means that everything worked perfectly, and you obtained all the product that could have been produced. Anyone who has tried to do something as simple as fill a salt shaker or add oil to a car’s engine without spilling knows how unlikely a 100% yield is. At the other extreme, a yield of 0% means that no product was obtained. A percent yield of 80%–90% is usually considered good to excellent; a yield of 50% is only fair. In part because of the problems and costs of waste disposal, industrial production facilities face considerable pressures to optimize the yields of products and make them as close to 100% as possible.